- Главная
- Разное
- Бизнес и предпринимательство
- Образование
- Развлечения
- Государство
- Спорт
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Религиоведение
- Черчение
- Физкультура
- ИЗО
- Психология
- Социология
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Геометрия
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Что такое findslide.org?
FindSlide.org - это сайт презентаций, докладов, шаблонов в формате PowerPoint.
Обратная связь
Email: Нажмите что бы посмотреть
Презентация на тему Oscillatory motion. The simple pendulum. (Lecture 1)
Содержание
- 2. Lecture 1Oscillatory motion. Simple harmonic motion. The simple pendulum. Damped harmonic oscillations.Driven harmonic oscillations.
- 3. Harmonic Motion of Object with Spring A block
- 4. x is displacement from equilibrium position.Restoring force
- 5. Simple Harmonic MotionAn object moves with simple
- 6. Mathematical Representation of Simple Harmonic MotionSo
- 7. A=const is the amplitude of the
- 8. The inverse of the period is the frequency f of the oscillations:
- 9. Then the velocity and the acceleration of a body in simple harmonic motion are:
- 10. Position vs timeVelocity vs time At any specified
- 11. Energy of the Simple Harmonic OscillatorAssuming that:no
- 12. The total mechanical energy of simple harmonic
- 13. Simple PendulumSimple pendulum consists of a particle-like
- 15. The period and frequency of a simple
- 16. Physical Pendulum If a hanging object oscillates about
- 17. Applying the rotational form of the second Newton’s law:The solution is:The period is
- 18. Damped Harmonic OscillationsIn many real systems, nonconservative
- 19. The solution for small b isWhen the
- 20. The angular frequency can be expressed through
- 21. underdamped oscillator: Rmax=bVmaxkA and b/(2m)>ω0 .
- 22. Driven Harmonic OscillationsA driven (or forced) oscillator
- 23. The forced oscillator vibrates at the frequency
- 24. ResonanceSo resonance happens when the driving force
- 25. Скачать презентацию
- 26. Похожие презентации
Lecture 1Oscillatory motion. Simple harmonic motion. The simple pendulum. Damped harmonic oscillations.Driven harmonic oscillations.




















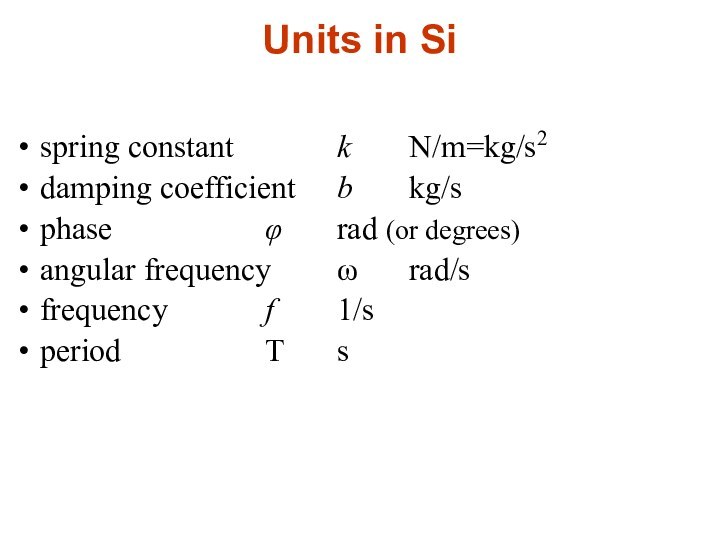
Слайд 3
Harmonic Motion of Object with Spring
A block attached
to a spring moving on a frictionless surface.
(a)
When the block is displaced to the right of equilibrium (x > 0), the force exerted by the spring acts to the left. (b) When the block is at its equilibrium position (x = 0), the force exerted by the spring is zero.
(c) When the block is displaced to the left of equilibrium (x < 0), the force exerted by the spring acts to the right.
So the force acts opposite to displacement.
Слайд 4
x is displacement from equilibrium position.
Restoring force is
given by Hook’s law:
Then we can obtain the acceleration:
That
is, the acceleration is proportional to the position of the block, and its direction is opposite the direction of the displacement from equilibrium.
Слайд 5
Simple Harmonic Motion
An object moves with simple harmonic
motion whenever its acceleration is proportional to its position
and is oppositely directed to the displacement from equilibrium.
Слайд 6
Mathematical Representation
of Simple Harmonic Motion
So the equation
for harmonic motion is:
We can denote angular frequency as:
Then:
Solution for this equation is:
Слайд 7
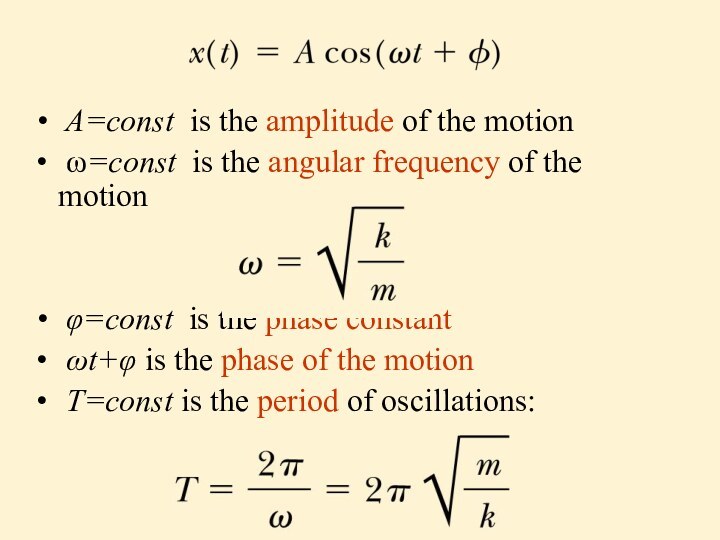
A=const is the amplitude of the motion
ω=const is the angular frequency of the motion
φ=const
is the phase constantωt+φ is the phase of the motion
T=const is the period of oscillations:
Слайд 10
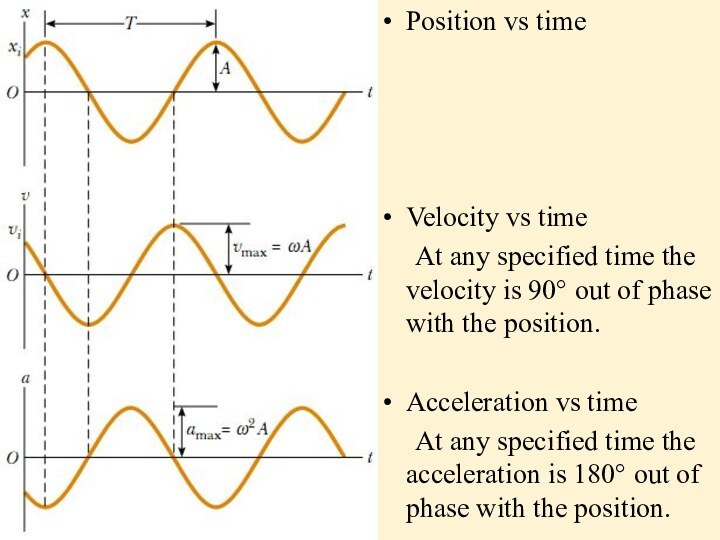
Position vs time
Velocity vs time
At any specified time
the velocity is 90° out of phase with the
position.Acceleration vs time
At any specified time the acceleration is 180° out of phase with the position.
Слайд 11
Energy of the Simple Harmonic Oscillator
Assuming that:
no friction
the
spring is massless
Then the kinetic energy of system spring-body
corresponds only to that of the body:The potential energy in the spring is:
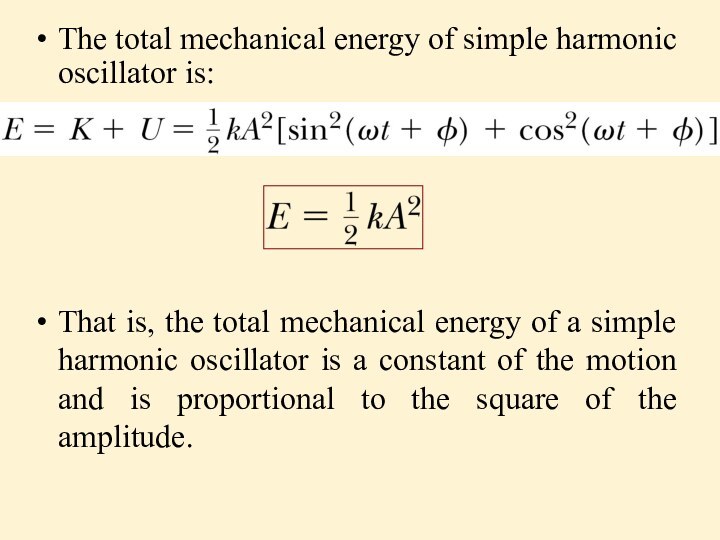
Слайд 12 The total mechanical energy of simple harmonic oscillator
is:
That is, the total mechanical energy of a simple
harmonic oscillator is a constant of the motion and is proportional to the square of the amplitude.
Слайд 13
Simple Pendulum
Simple pendulum consists of a particle-like bob
of mass m suspended by a light string of
length L that is fixed at the upper end.The motion occurs in the vertical plane and is driven by the gravitational force.
When Θ is small, a simple pendulum oscillates in simple harmonic motion about the equilibrium position Θ = 0. The restoring force is -mgsinΘ, the component of the gravitational force tangent to the arc.
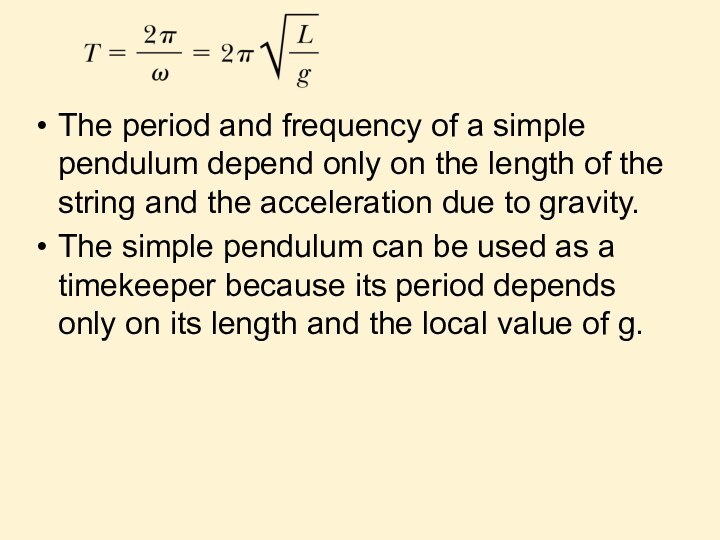
Слайд 15 The period and frequency of a simple pendulum
depend only on the length of the string and
the acceleration due to gravity.The simple pendulum can be used as a timekeeper because its period depends only on its length and the local value of g.
Слайд 16
Physical Pendulum
If a hanging object oscillates about a
fixed axis that does not pass through its center
of mass and the object cannot be approximated as a point mass, we cannot treat the system as a simple pendulum. In this case the system is called a physical pendulum.
Слайд 18
Damped Harmonic Oscillations
In many real systems, nonconservative forces,
such as friction, retard the motion. Consequently, the mechanical
energy of the system diminishes in time, and the motion is damped. The retarding force can be expressed as R=-bv (b=const is the damping coefficient) and the restoring force of the system is -kx then:
Слайд 19
The solution for small b is
When the retarding
force is small, the oscillatory character of the motion
is preserved but the amplitude decreases in time, with the result that the motion ultimately ceases.Слайд 20 The angular frequency can be expressed through ω0=(k/m)1/2
– the natural frequency of the system (the undamped
oscillator):Слайд 21 underdamped oscillator: Rmax=bVmax
damped oscillator: when b has critical value bc= 2mω0
. System does not oscillate, just returns to the equilibrium position.overdamped oscillator: Rmax=bVmax>kA and b/(2m)>ω0 . System does not oscillate, just returns to the equilibrium position.
Слайд 22
Driven Harmonic Oscillations
A driven (or forced) oscillator is
a damped oscillator under the influence of an external
periodical force F(t)=F0sin(ωt). The second Newton’s law for forced oscillator is:The solution of this equation is:
Слайд 23 The forced oscillator vibrates at the frequency of
the driving force
The amplitude of the oscillator is constant
for a given driving force. For small damping, the amplitude is large when the frequency of the driving force is near the natural frequency of oscillation, or when ω≈ω0.
The dramatic increase in amplitude near the natural frequency is called resonance, and the natural frequency ω0 is also called the resonance frequency of the system.
Слайд 24
Resonance
So resonance happens when the driving force frequency
is close to the natural frequency of the system:
ω≈ω0. At resonance the amplitude of the driven oscillations is the largest.In fact, if there were no damping (b = 0), the amplitude would become infinite when ω=ω0. This is not a realistic physical situation, because it corresponds to the spring being stretched to infinite length. A real spring will snap rather than accept an infinite stretch; in other words, some for of damping will ultimately occur, But it does illustrate that, at resonance, the response of a harmonic system to a driving force can be catastrophically large.





























