- Главная
- Разное
- Бизнес и предпринимательство
- Образование
- Развлечения
- Государство
- Спорт
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Религиоведение
- Черчение
- Физкультура
- ИЗО
- Психология
- Социология
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Геометрия
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Что такое findslide.org?
FindSlide.org - это сайт презентаций, докладов, шаблонов в формате PowerPoint.
Обратная связь
Email: Нажмите что бы посмотреть
Презентация на тему Physics 1 for KMA
Содержание
- 2. Lecture 4 Rotation of rigid bodies. Angular momentum and torque.Properties of fluids.Flotation.Bernulli equation.
- 3. Rotation of Rigid BodiesWhen a rigid object
- 4. Radians
- 5. Angular kinematicsAngular displacement:Instantaneous angular speed:Instantaneous angular acceleration:
- 6. Angular and linear quantitiesEvery particle of the
- 7. Total linear accelerationTangential acceleration is perpendicular to
- 8. Angular velocityAngular velocity is a vector.
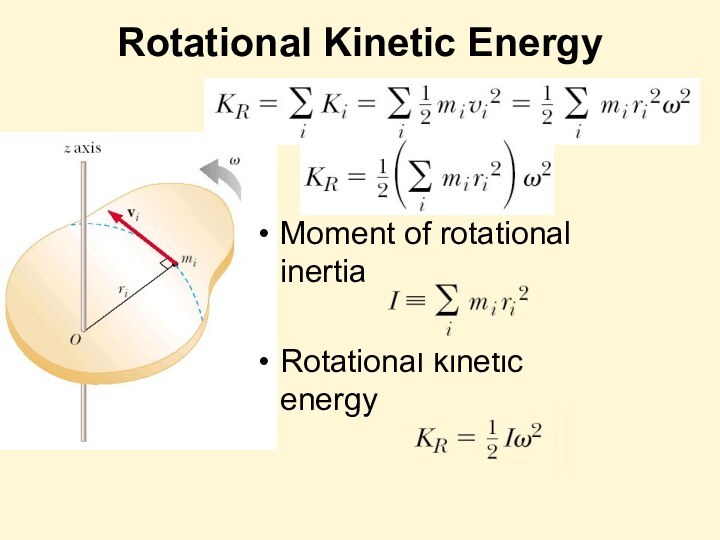
- 9. Rotational Kinetic Energy Moment of rotational inertiaRotational kinetic energy
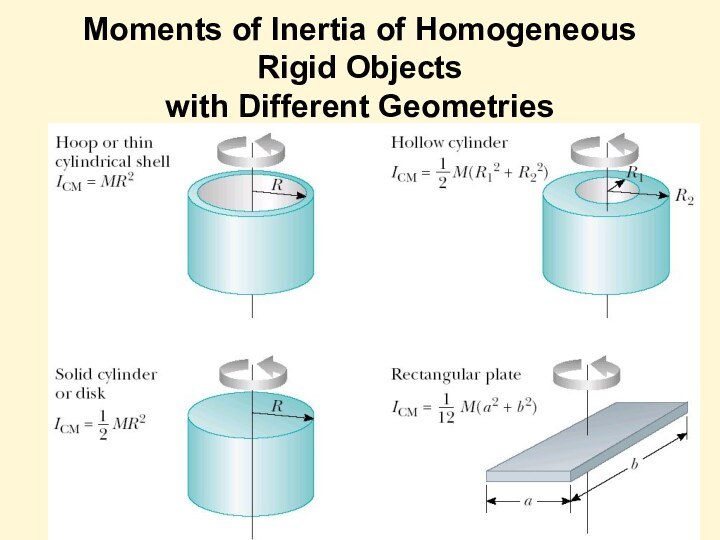
- 10. Calculations of Moments of Inertia
- 11. Uniform Thin Hoop
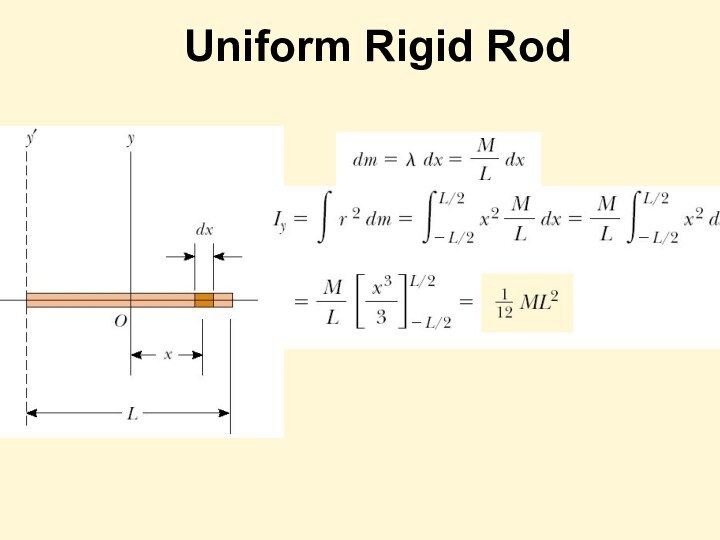
- 12. Uniform Rigid Rod
- 13. Uniform Solid Cylinder
- 14. Moments of Inertia of Homogeneous Rigid Objects with Different Geometries
- 16. Parallel-axis theoremSuppose the moment of inertia about
- 18. Torque When a force is exerted on a
- 19. The force F has a greater rotating
- 20. The force F1 tends to rotate the
- 21. Torque is not Force Torque is not
- 22. Rotational DynamicsLet’s add
- 23. Rotational analogue of Newton’s second lawQuantity L
- 24. Net External Torque The net external torque acting
- 25. Angular Momentum of a Rotating Rigid ObjectAngular
- 26. Angular acceleration
- 27. The Law of Angular Momentum ConservationThe total
- 28. Change in internal structure of a rotating body can result in change of its angular velocity.
- 29. When a rotating skater pulls his hands towards his body he spins faster.
- 30. Three Laws of Conservation for an Isolated
- 31. Work-Kinetic Theory for RotationsSimilarly to linear motion:
- 32. The net work done by external forces
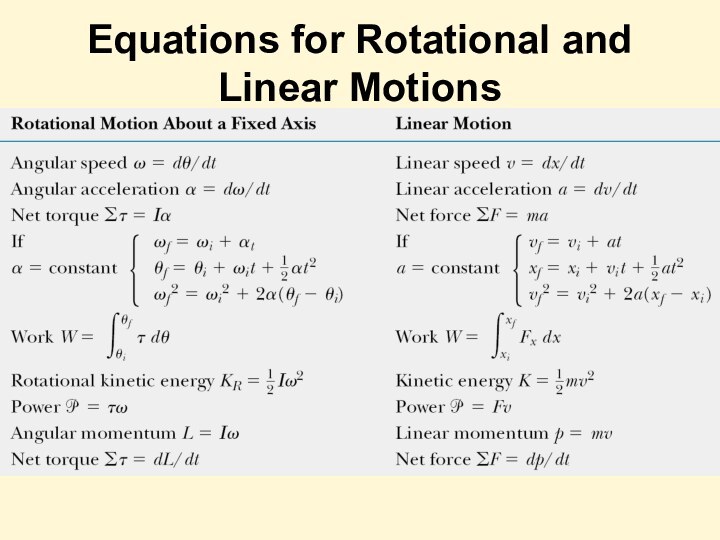
- 33. Equations for Rotational and Linear Motions
- 34. Gyroscope One typical type of gyroscope is made
- 35. At high speeds, the gyroscope exhibits extraordinary
- 36. If a gyroscope is tipped, the gimbals
- 37. Precession of Spinning Wheel
- 38. Fluids and liquids
- 39. Relative densityRelative density or specific gravity is
- 41. Specific volume of a substance is the
- 42. Pressure
- 43. ManometerThe difference in fluid height in a liquid column manometer is proportional to the pressure difference.P1-P2=ρgh
- 44. Static Fluid PressurePstatic fluid = ρgh
- 45. Pressure ThrustThrust is a total force in
- 46. Atmospheric PressureThe surface of the earth is
- 47. Atmospheric constituents
- 48. Barometer
- 49. Aneroid barometerAn aneroid barometeru ses a small,
- 51. The Barometric Formulaμair=28.9644 g/molmair= μair/Na
- 52. Pascal's PrinciplePressure exerted anywhere in a confined
- 54. Hydraulic Press
- 55. Lift pump The lift pump, also known as
- 56. Force pump The force pump, also known as
- 57. Rotary PumpsRotary vane pumpScroll pump
- 58. Height limitation Total Dynamic Head (TDH) is the
- 59. Total Dynamic Height TDH = Static Height +
- 60. Viscosity The resistance to flow of a fluid
- 61. Experimentally, under conditions of laminar flow, the
- 63. Drag force due viscosity In a viscous fluid,
- 64. Effect of Temperature on Viscosity The temperature
- 65. Liquid DampingDamping is an effect that reduces
- 68. Buoyancy Buoyancy arises from the fact that
- 69. Archimedes' PrincipleThe buoyant force on a submerged
- 70. Hydrometer A hydrometer is an instrument used
- 72. Determine, what liquid is denser?
- 73. This liquid is lighter.This liquid is denser.This liquid is lighter.
- 74. Fluid Kinetic EnergyThe kinetic energy of a
- 75. Fluid Potential EnergyThe potential energy of a
- 76. Bernoulli Equation
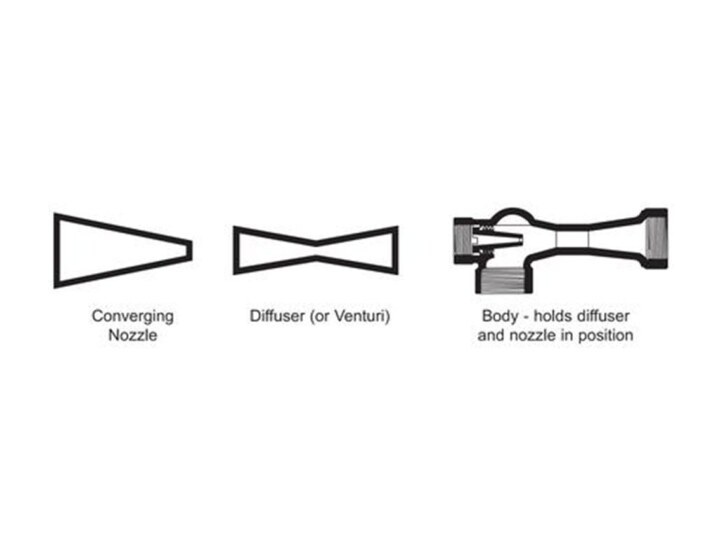
- 77. Venturi meter The Venturi effect is the reduction
- 78. Venturi effect Q is volumetric flow rateSo Venturi meter can be used to measure the flow rate.
- 79. Water EductorLiquid Jet Eductors use the kinetic
- 81. Скачать презентацию
- 82. Похожие презентации
Lecture 4 Rotation of rigid bodies. Angular momentum and torque.Properties of fluids.Flotation.Bernulli equation.



































































Слайд 3
Rotation of Rigid Bodies
When a rigid object is
rotating about a fixed axis, every particle of the
object rotates through the same angle in a given time interval and has the same angular speed and the same angular acceleration. So the rotational motion of the entire rigid object as well as individual particles in the object can be described by three angles. Using these three angles we can greatly simplify the analysis of rigid-object rotation.
Слайд 5
Angular kinematics
Angular displacement:
Instantaneous angular speed:
Instantaneous angular acceleration:
Слайд 6
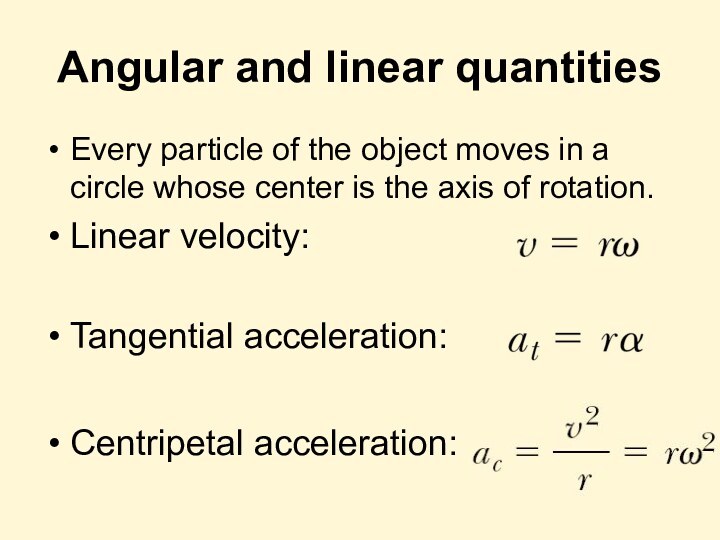
Angular and linear quantities
Every particle of the object
moves in a circle whose center is the axis
of rotation.Linear velocity:
Tangential acceleration:
Centripetal acceleration:
Слайд 7
Total linear acceleration
Tangential acceleration is perpendicular to the
centripetal one, so the magnitude of total linear acceleration
is
Слайд 8
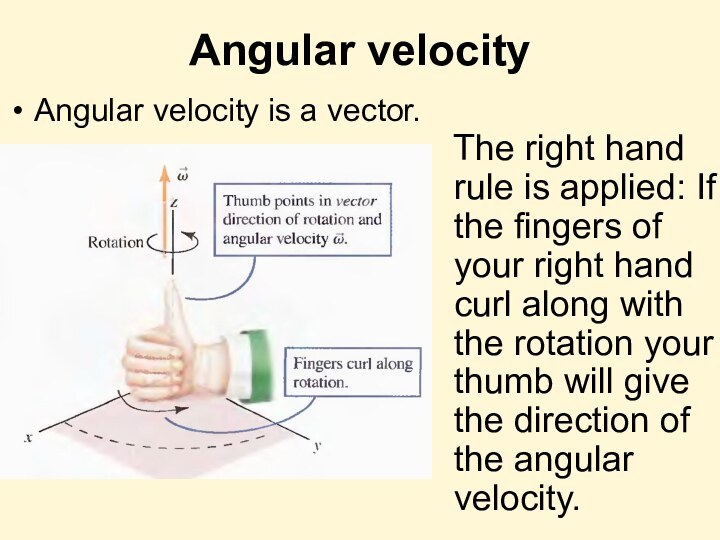
Angular velocity
Angular velocity is a vector.
The right hand rule is applied: If the fingers
of your right hand curl along with the rotation your thumb will give the direction of the angular velocity.
Слайд 16
Parallel-axis theorem
Suppose the moment of inertia about an
axis through the center of mass of an object
is ICM. Then the moment of inertia about any axis parallel to and a distance D away from this axis is
Слайд 18
Torque
When a force is exerted on a rigid
object pivoted about an axis, the object tends to
rotate about that axis. The tendency of a force to rotate an object about some axis is measured by a vector quantity called torque τ (Greek tau).
Слайд 19
The force F has a greater rotating tendency
about axis O as F increases and as the
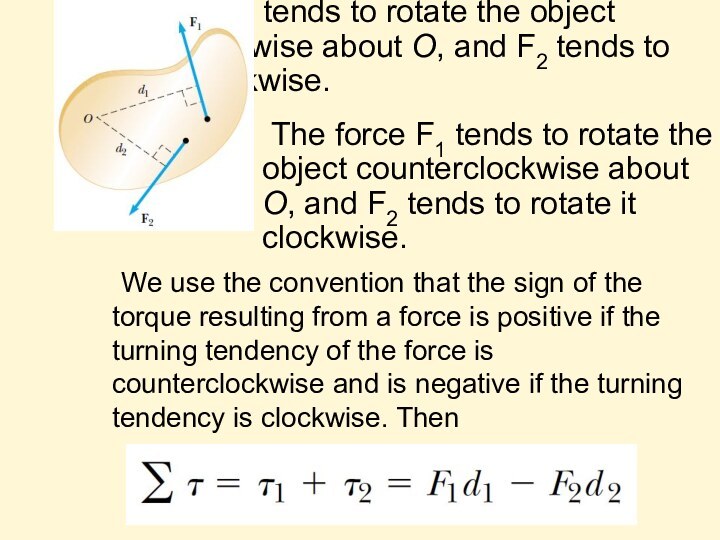
moment arm d increases. The component F sinφ tends to rotate the wrench about axis O.Слайд 20 The force F1 tends to rotate the object
counterclockwise about O, and F2 tends to rotate it
clockwise. We use the convention that the sign of the torque resulting from a force is positive if the turning tendency of the force is counterclockwise and is negative if the turning tendency is clockwise. Then
The force F1 tends to rotate the object counterclockwise about O, and F2 tends to rotate it clockwise.
Слайд 21
Torque is not Force
Torque is not Work
Torque should
not be confused with force. Forces can cause a
change in linear motion, as described by Newton’s second law. Forces can also cause a change in rotational motion, but the effectiveness of the forces in causing this change depends on both the forces and the moment arms of the forces, in the combination that we call torque. Torque has units of force times length—newton · meters in SI units—and should be reported in these units.Do not confuse torque and work, which have the same units but are very different concepts.
Слайд 23
Rotational analogue of Newton’s second law
Quantity L is
an instantaneous angular momentum.
The torque acting on a particle
is equal to the time rate of change of the particle’s angular momentum.
Слайд 24
Net External Torque
The net external torque acting on
a system about some axis passing through an origin
in an inertial frame equals the time rate of change of the total angular momentum of the system about that origin:
Слайд 25
Angular Momentum of a Rotating Rigid Object
Angular momentum
for each particle of an object:
Angular momentum for the
whole object:Thus:
Слайд 27
The Law of Angular Momentum Conservation
The total angular
momentum of a system is constant if the resultant
external torque acting on the system is zero, that is, if the system is isolated.
Слайд 28
Change in internal structure of a rotating body
can result in change of its angular velocity.
Слайд 30
Three Laws of Conservation for an Isolated System
Full
mechanical energy, linear momentum and angular momentum of an
isolated system remain constant.
Слайд 32
The net work done by external forces in
rotating a symmetric rigid object about a fixed axis
equals the change in the object’s rotational energy.
Слайд 34
Gyroscope
One typical type of gyroscope is made by
suspending a relatively massive rotor inside three rings called
gimbals. Mounting each of these rotors on high quality bearing surfaces insures that very little torque can be exerted on the inside rotor.
Слайд 35
At high speeds, the gyroscope exhibits extraordinary stability
of balance and maintains the direction of the high
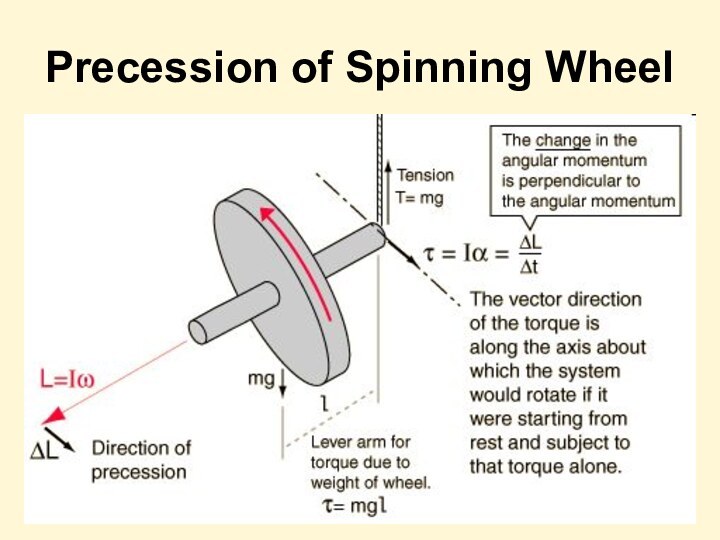
speed rotation axis of its central rotor. The implication of the conservation of angular momentum is that the angular momentum of the rotor maintains not only its magnitude, but also its direction in space in the absence of external torque. The classic type gyroscope finds application in gyro-compasses.Слайд 36 If a gyroscope is tipped, the gimbals will
try to reorient to keep the spin axis of
the rotor in the same direction. If released in this orientation, the gyroscope will precess in the direction shown because of the torque exerted by gravity on the gyroscope.
Слайд 39
Relative density
Relative density or specific gravity is the
ratio of the density of a substance to the
density of a given reference material. Specific gravity usually means relative density with respect to water.If the reference material is water then a substance with a relative density (or specific gravity) less than 1 will float in water. For example, an ice cube, with a relative density of about 0.91, will float. A substance with a relative density greater than 1 will sink.
Слайд 41 Specific volume of a substance is the ratio
of the substance's volume to its mass. It is
the reciprocal of density and is an intrinsic property of matter:
Слайд 43
Manometer
The difference in fluid height in a liquid
column manometer is proportional to the pressure difference.
P1-P2=ρgh
Слайд 44
Static Fluid Pressure
Pstatic fluid = ρgh where ρ
= m/V = fluid density
g = gravitational acceleration
h =
depth of fluidThe pressure exerted by a static fluid depends only upon the depth of the fluid, the density of the fluid, and the acceleration of gravity
Слайд 45
Pressure Thrust
Thrust is a total force in a
particular direction. The unit of thrust, therefore is the
same as that of force: Newtons (N). Pressure is the force or thrust applied per unit area.F=P·A
Слайд 46
Atmospheric Pressure
The surface of the earth is at
the bottom of an atmospheric sea. The standard atmospheric
pressure is measured in various units:1 atmosphere = 760 mmHg = 101.3 KPa
The bar is a unit of pressure defined as 100 kilopascals. It is about equal to the atmospheric pressure on Earth at sea level.
The unit mmHg is often called torr, particularly in vacuum applications: 760 mmHg = 760 torr
Слайд 49
Aneroid barometer
An aneroid barometeru ses a small, flexible
metal box called an aneroid cell (capsule), which is
made from an alloy of beryllium and copper. The evacuated capsule (or usually more capsules) is prevented from collapsing by a strong spring. Small changes in external air pressure cause the cell to expand or contract. This expansion and contraction drives mechanical levers such that the tiny movements of the capsule are amplified and displayed on the face of the aneroid barometer.
Слайд 52
Pascal's Principle
Pressure exerted anywhere in a confined incompressible
fluid is transmitted equally in all directions throughout the
fluid such that the pressure ratio (initial difference) remains the same.
Слайд 55
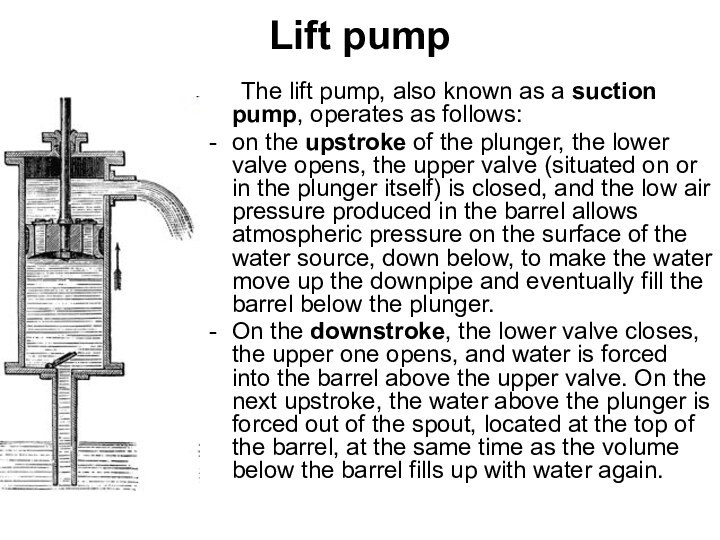
Lift pump
The lift pump, also known as a
suction pump, operates as follows:
on the upstroke of
the plunger, the lower valve opens, the upper valve (situated on or in the plunger itself) is closed, and the low air pressure produced in the barrel allows atmospheric pressure on the surface of the water source, down below, to make the water move up the downpipe and eventually fill the barrel below the plunger.On the downstroke, the lower valve closes, the upper one opens, and water is forced into the barrel above the upper valve. On the next upstroke, the water above the plunger is forced out of the spout, located at the top of the barrel, at the same time as the volume below the barrel fills up with water again.
Слайд 56
Force pump
The force pump, also known as a
pressure pump, operates as follows:
on the upstroke of
the plunger, the outlet or delivery valve is closed and the inlet valve opens. The low air pressure produced in the barrel causes the water below to move up the downpipe and eventually fill the barrel. On the downstroke, the inlet valve closes, the outlet valve opens, and the water is forced out via the outlet pipe, which is located at the bottom of the barrel.
Слайд 58
Height limitation
Total Dynamic Head (TDH) is the total
equivalent height that a fluid is to be pumped,
taking into account friction losses in the pipe.
Слайд 59
Total Dynamic Height
TDH = Static Height + Static
Lift + Friction Loss
Static Height is the maximum height
reached by the pipe after the pump (also known as the 'discharge head').Static Lift is the height the water will rise before arriving at the pump (also known as the suction head).
Friction Loss - in any real moving fluid, energy is dissipated due to friction; turbulence dissipates even more energy for high Reynolds number flows. Friction loss is divided into two main categories, "major losses" associated with energy loss per length of pipe, and "minor losses" associated with bends, fittings, valves, etc.
Слайд 60
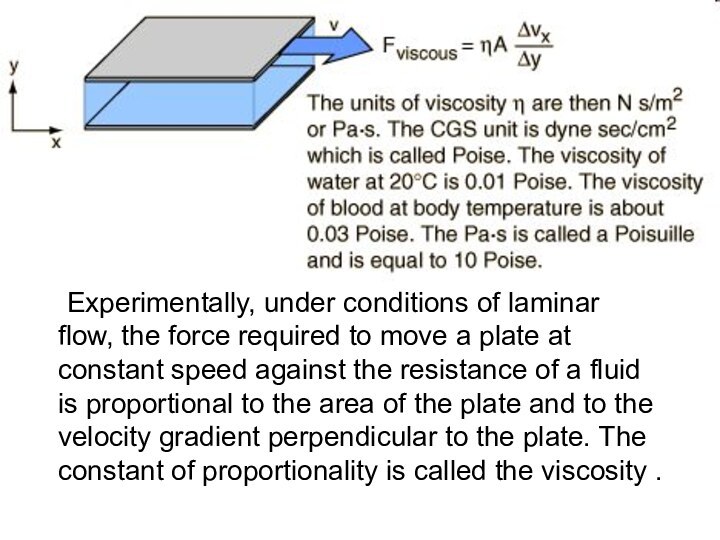
Viscosity
The resistance to flow of a fluid and
the resistance to the movement of an object through
a fluid are usually stated in terms of the viscosity of the fluid.
Слайд 61
Experimentally, under conditions of laminar flow, the force
required to move a plate at constant speed against
the resistance of a fluid is proportional to the area of the plate and to the velocity gradient perpendicular to the plate. The constant of proportionality is called the viscosity .
Слайд 63
Drag force due viscosity
In a viscous fluid, a
boundary layer is formed. This causes a net drag
due to skin friction. Further, because the ideal pressure now acts on the boundary layer, as opposed to the ship, and the boundary layer grows along the length of the ship, the net opposing forces are greater than the net supporting forces. This further adds to the resistance.
Слайд 64
Effect of Temperature on Viscosity
The temperature dependence
of liquid viscosity is the phenomenon by which liquid
viscosity tends to decrease (or, alternatively, its fluidity tends to increase) as its temperature increases. here η0 and b are constants.
This is an empirical model that usually works for a limited range of temperatures.
Слайд 65
Liquid Damping
Damping is an effect that reduces the
amplitude of oscillations in an oscillatory system
Fluid viscous damping
is a way to add energy dissipation to the lateral system of a building structure. A fluid viscous damper dissipates energy by pushing fluid through an orifice, producing a damping pressure which creates a force. These damping forces are 90 degrees out of phase with the displacement driven forces in the structure. This means that the damping force does not significantly increase the seismic loads for a comparable degree of structural deformation.
Слайд 68
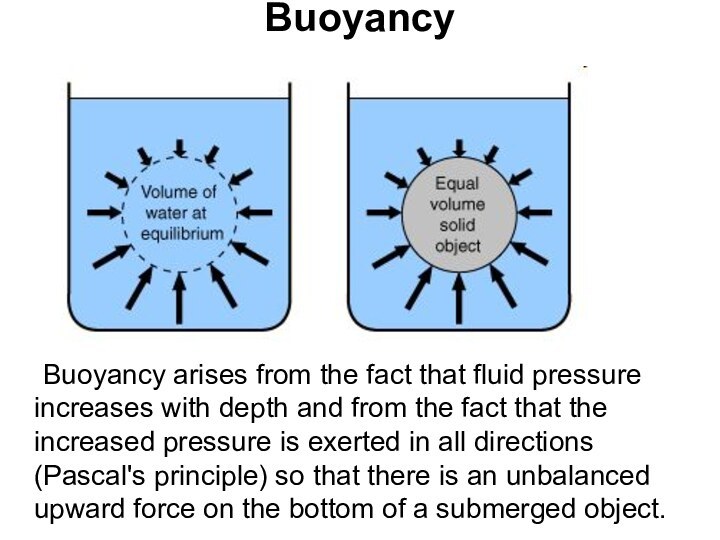
Buoyancy
Buoyancy arises from the fact that fluid pressure
increases with depth and from the fact that the
increased pressure is exerted in all directions (Pascal's principle) so that there is an unbalanced upward force on the bottom of a submerged object.
Слайд 69
Archimedes' Principle
The buoyant force on a submerged object
is equal to the weight of the fluid displaced.
The upward thrust which the surrounding fluid exerts on an object is referred to as the force of buoyancy.
Слайд 70
Hydrometer
A hydrometer is an instrument used to
measure the specific gravity (or relative density) of liquids;
that is, the ratio of the density of the liquid to the density of water.A hydrometer is usually made of glass and consists of a cylindrical stem and a bulb weighted with mercury or lead shot to make it float upright. The liquid to be tested is poured into a tall container, often a graduated cylinder, and the hydrometer is gently lowered into the liquid until it floats freely. The point at which the surface of the liquid touches the stem of the hydrometer is noted. Hydrometers usually contain a scale inside the stem, so that the specific gravity can be read directly. A variety of scales exist, and are used depending on the context.
Hydrometers may be calibrated for different uses, such as a lactometer for measuring the density (creaminess) of milk, a saccharometer for measuring the density of sugar in a liquid, or an alcoholometer for measuring higher levels of alcohol in spirits.
Слайд 74
Fluid Kinetic Energy
The kinetic energy of a moving
fluid is more useful in applications like the Bernoulli
equation when it is expressed as kinetic energy per unit volume
Слайд 75
Fluid Potential Energy
The potential energy of a moving
fluid is more useful in applications like the Bernoulli
equation when is expressed as potential energy per unit volume
Слайд 77
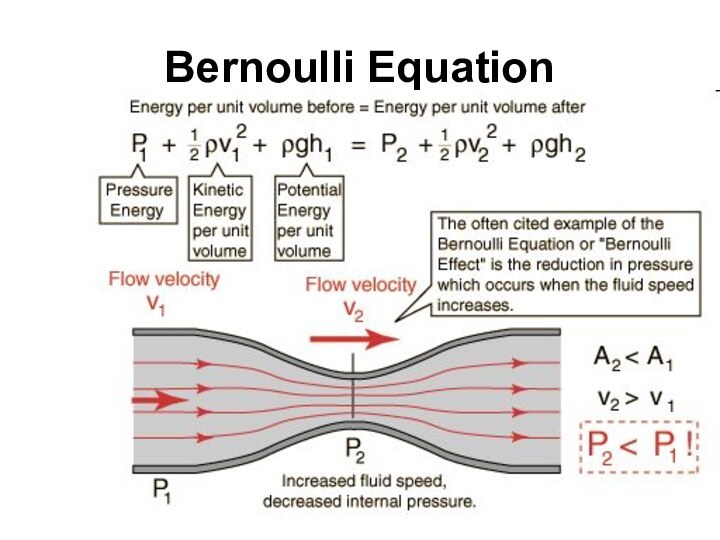
Venturi meter
The Venturi effect is the reduction in
fluid pressure that results when a fluid flows through
a constricted section of pipe.The Venturi effect is named after Giovanni Battista Venturi (1746–1822), an Italian physicist.





























