Слайд 2
Irreversibility of processes
There exist many processes that are
irreversible:
the net transfer of energy by heat is always
from the warmer object to the cooler object, never from the cooler to the warmer
an oscillating pendulum eventually comes to rest because of collisions with air molecules and friction. The mechanical energy of the system converted to internal energy in the air, the pendulum, and the suspension; the reverse conversion of energy never occurs.
Слайд 3
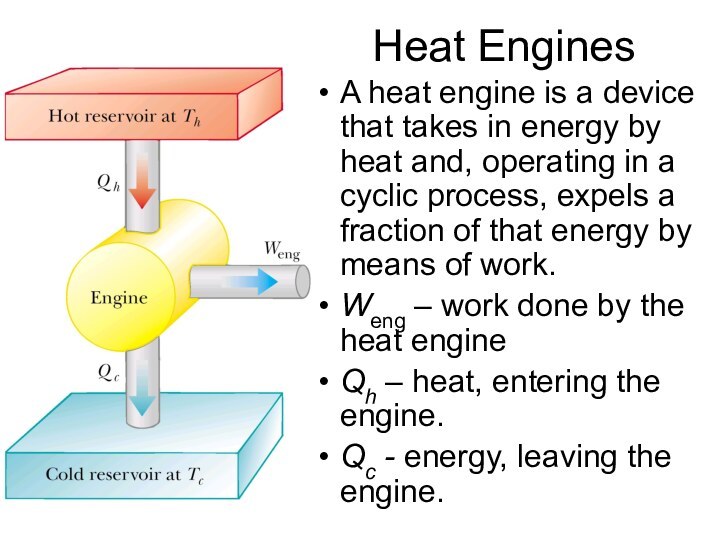
Heat Engines
A heat engine is a device that
takes in energy by heat and, operating in a
cyclic process, expels a fraction of that energy by means of work.
Weng – work done by the heat engine
Qh – heat, entering the engine.
Qc - energy, leaving the engine.
Слайд 4
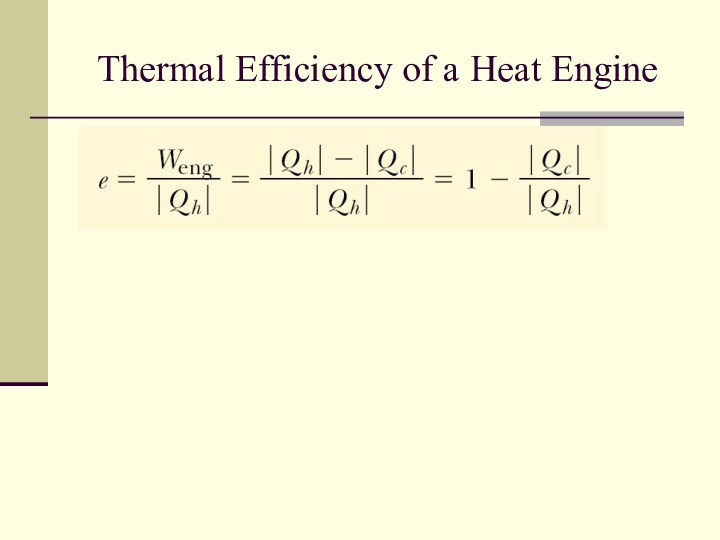
Thermal Efficiency of a Heat Engine
Слайд 5
Heat Pumps or Refrigerators
In a heat engine a
fraction of heat from the hot reservoir is used
to perform work.
In a refrigerator or a heat pump work is used to take heat from the cold reservoir and directed to the hot reservoir.
Слайд 6
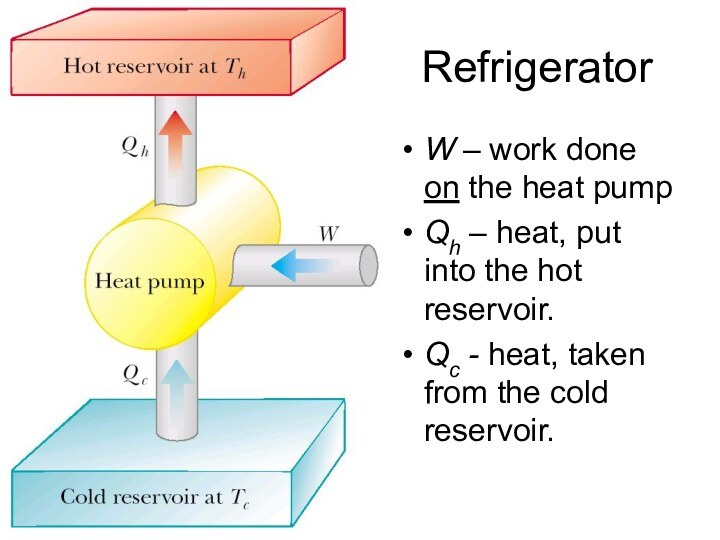
Refrigerator
W – work done on the heat pump
Qh – heat, put into the hot reservoir.
Qc
- heat, taken from the cold reservoir.
Слайд 7
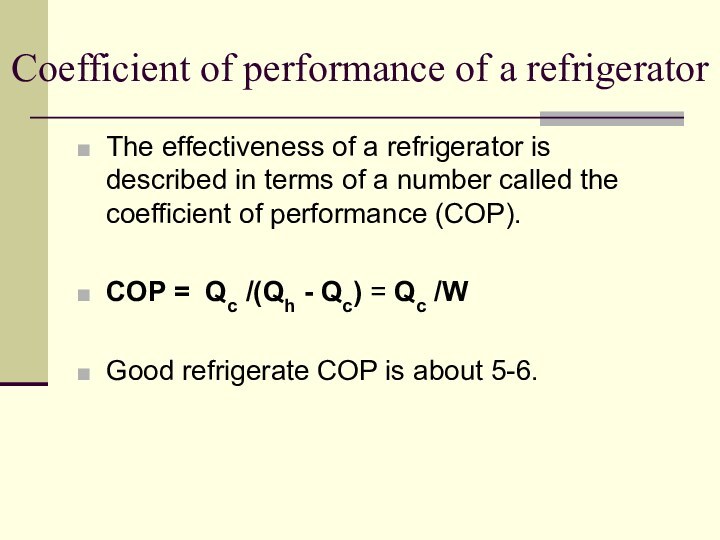
Coefficient of performance of a refrigerator
The effectiveness of
a refrigerator is described in terms of a number
called the coefficient of performance (COP).
COP = Qc /(Qh - Qc) = Qc /W
Good refrigerate COP is about 5-6.
Слайд 8
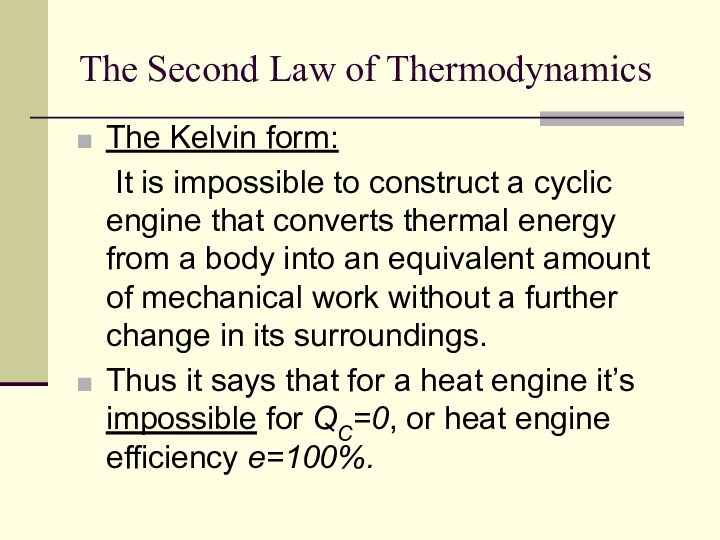
The Second Law of Thermodynamics
The Kelvin form:
It
is impossible to construct a cyclic engine that converts
thermal energy from a body into an equivalent amount of mechanical work without a further change in its surroundings.
Thus it says that for a heat engine it’s impossible for QC=0, or heat engine efficiency e=100%.
Слайд 9
The Second Law of Thermodynamics
The Clausius form:
It is
impossible to construct a cyclic engine which only effect
is to transfer thermal energy from a colder body to a hotter body.
Thus for refrigerator it’s impossible that W=0, or COP = ∞.
Слайд 10
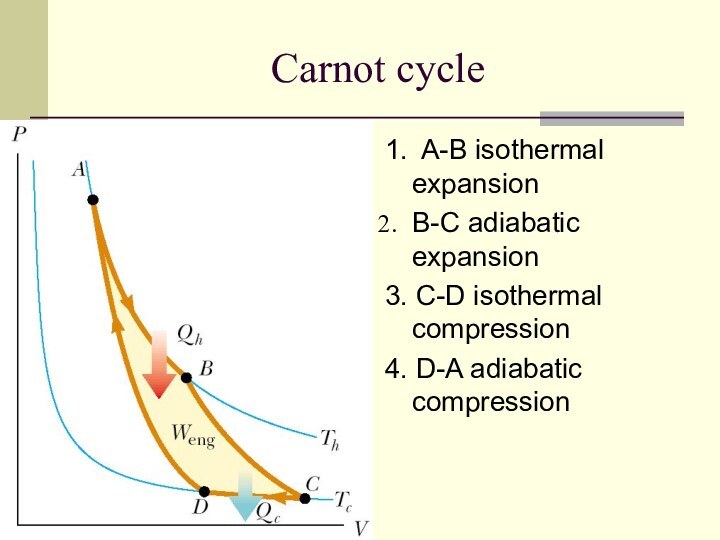
Carnot cycle
1. A-B isothermal expansion
B-C adiabatic expansion
3. C-D isothermal
compression
4. D-A adiabatic compression
Слайд 11
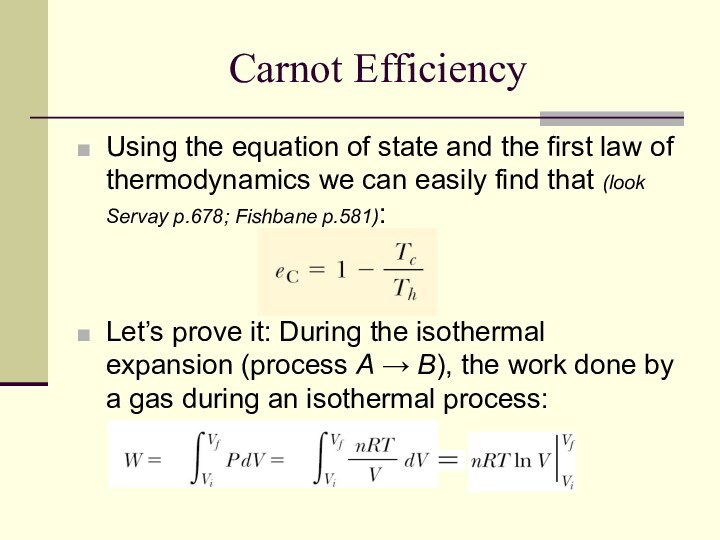
Carnot Efficiency
Using the equation of state and the
first law of thermodynamics we can easily find that
(look Servay p.678; Fishbane p.581):
Let’s prove it: During the isothermal expansion (process A → B), the work done by a gas during an isothermal process:
Слайд 12
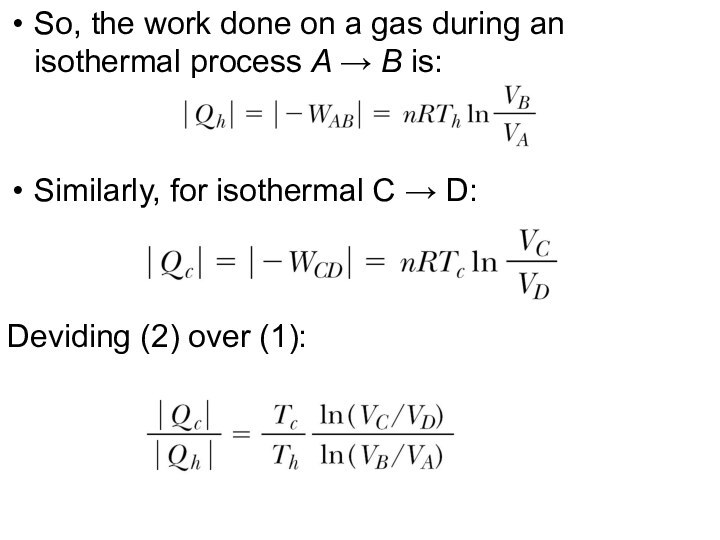
So, the work done on a gas during
an isothermal process A → B is:
(1)
Similarly, for isothermal
C → D:
(2)
Deviding (2) over (1):
(3)
Слайд 13
For adiabatic processes:
So, statement (3) gives us:
Слайд 14
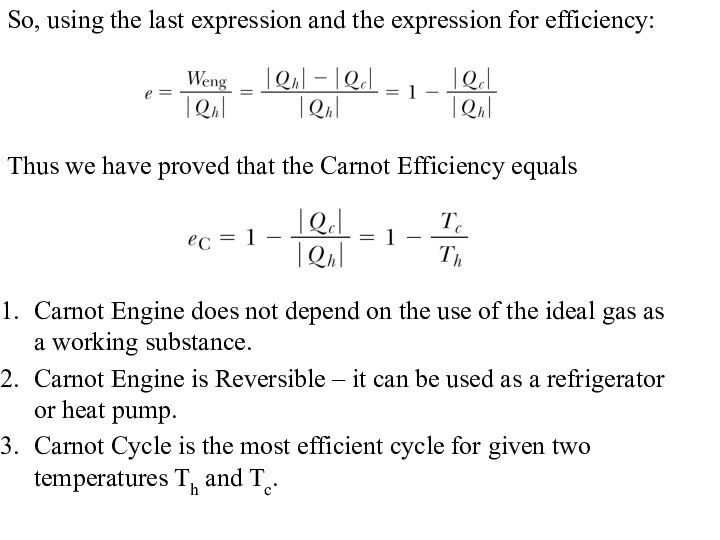
So, using the last expression and the expression
for efficiency:
Thus we have proved that the Carnot Efficiency
equals
Carnot Engine does not depend on the use of the ideal gas as a working substance.
Carnot Engine is Reversible – it can be used as a refrigerator or heat pump.
Carnot Cycle is the most efficient cycle for given two temperatures Th and Tc.
Слайд 15
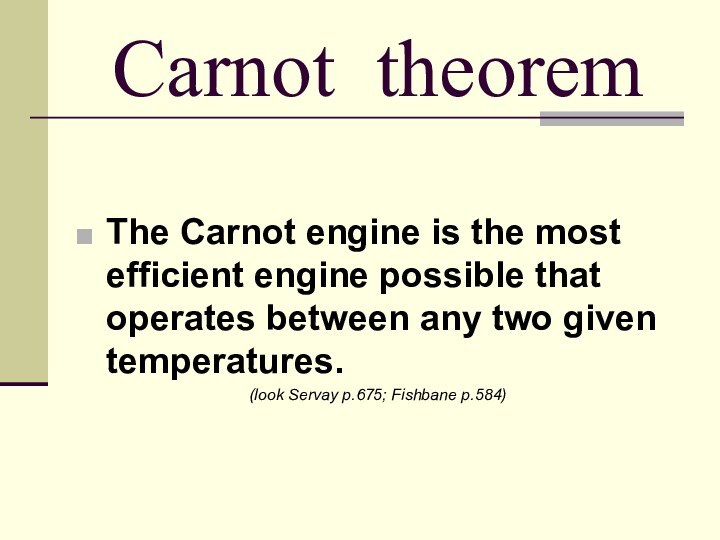
Carnot theorem
The Carnot engine is the most efficient
engine possible that operates between any two given temperatures.
(look Servay p.675; Fishbane p.584)
Слайд 16
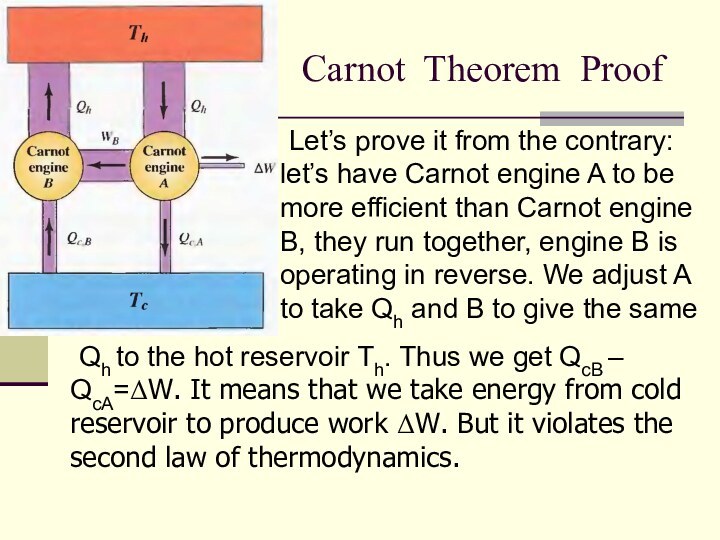
Carnot Theorem Proof
Let’s prove it from the contrary:
let’s have Carnot engine A to be more efficient
than Carnot engine B, they run together, engine B is operating in reverse. We adjust A to take Qh and B to give the same
Qh to the hot reservoir Th. Thus we get QcB – QcA=ΔW. It means that we take energy from cold reservoir to produce work ΔW. But it violates the second law of thermodynamics.
Слайд 17
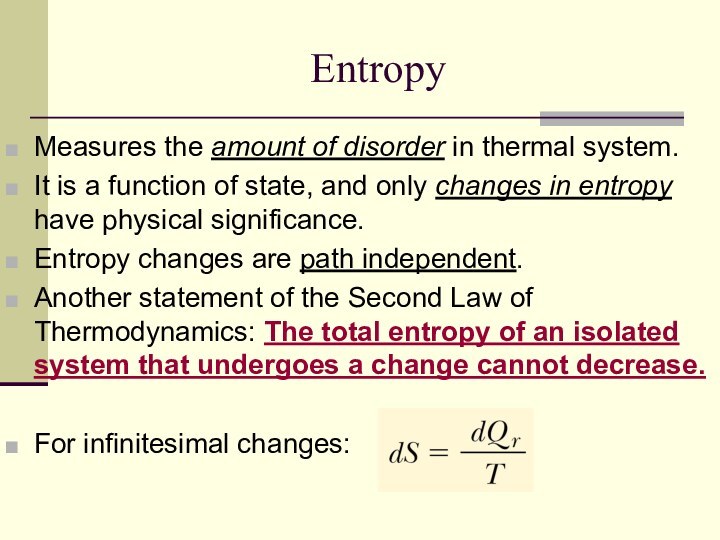
Entropy
Measures the amount of disorder in thermal system.
It
is a function of state, and only changes in
entropy have physical significance.
Entropy changes are path independent.
Another statement of the Second Law of Thermodynamics: The total entropy of an isolated system that undergoes a change cannot decrease.
For infinitesimal changes:
Слайд 18
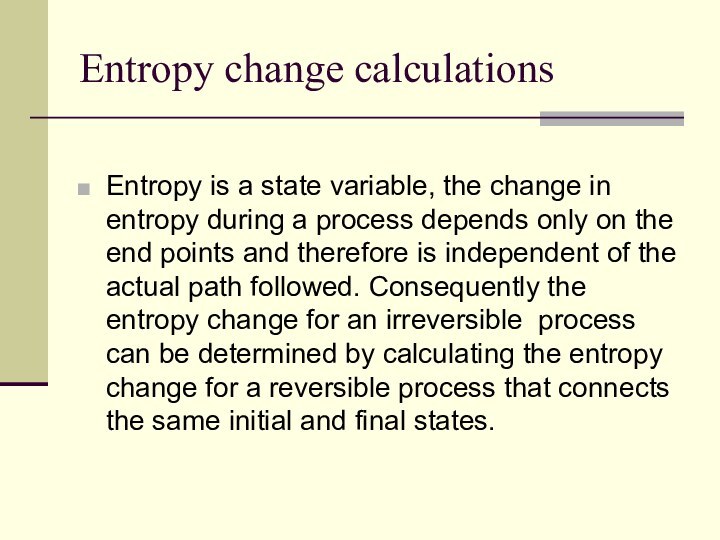
Entropy change calculations
Entropy is a state variable, the
change in entropy during a process depends only on
the end points and therefore is independent of the actual path followed. Consequently the entropy change for an irreversible process can be determined by calculating the entropy change for a reversible process that connects the same initial and final states.
Слайд 19
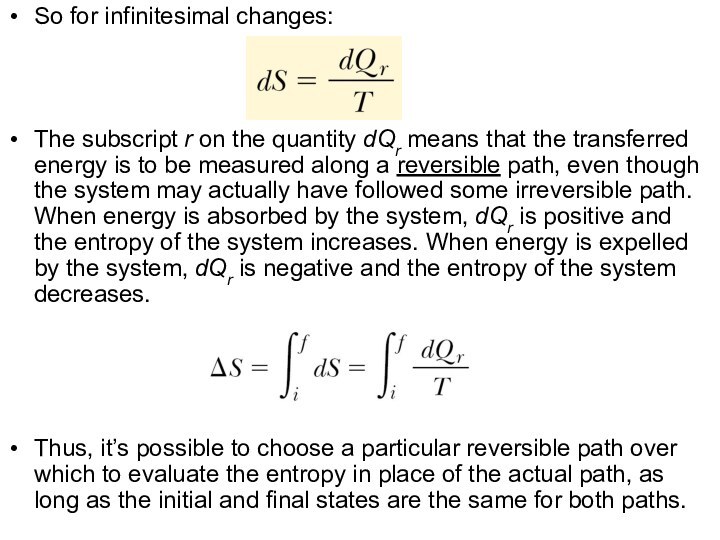
So for infinitesimal changes:
The subscript r on the
quantity dQr means that the transferred energy is to
be measured along a reversible path, even though the system may actually have followed some irreversible path. When energy is absorbed by the system, dQr is positive and the entropy of the system increases. When energy is expelled by the system, dQr is negative and the entropy of the system decreases.
Thus, it’s possible to choose a particular reversible path over which to evaluate the entropy in place of the actual path, as long as the initial and final states are the same for both paths.
Слайд 20
Change of Entropy in a Carnot Cycle
Carnot engine
operates between the temperatures Tc and Th. In one
cycle, the engine takes in energy Qh from the hot reservoir and expels energy Qc to the cold reservoir. These energy transfers occur only during the isothermal portions of the Carnot cycle thus the constant temperature can be brought out in front of the integral sign in expression
Thus, the total change in entropy for one cycle is
Слайд 21
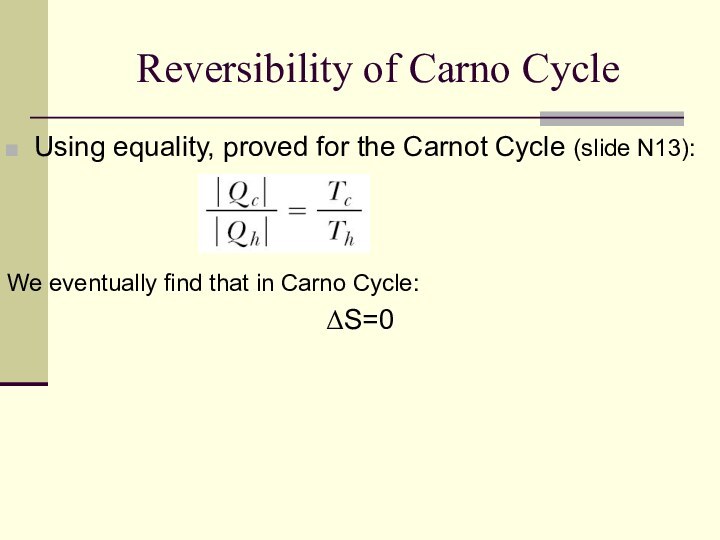
Reversibility of Carno Cycle
Using equality, proved for the
Carnot Cycle (slide N13):
We eventually find that in Carno
Cycle:
ΔS=0
Слайд 22
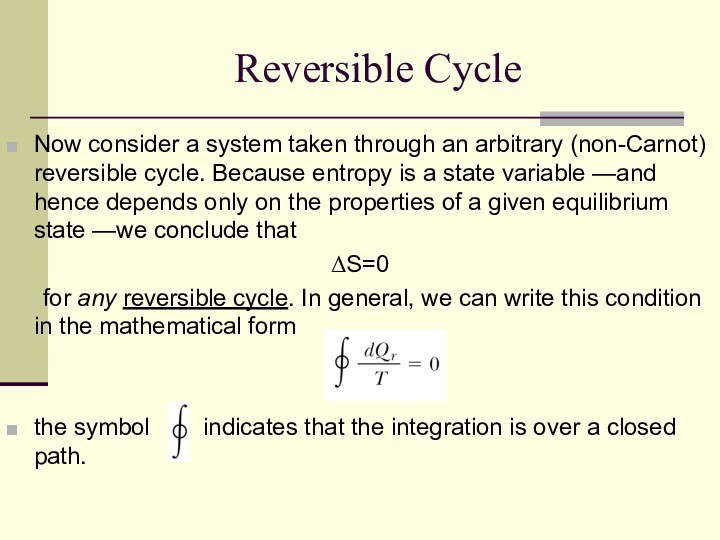
Reversible Cycle
Now consider a system taken through an
arbitrary (non-Carnot) reversible cycle. Because entropy is a state
variable —and hence depends only on the properties of a given equilibrium state —we conclude that
ΔS=0
for any reversible cycle. In general, we can write this condition in the mathematical form
the symbol indicates that the integration is over a closed path.
Слайд 23
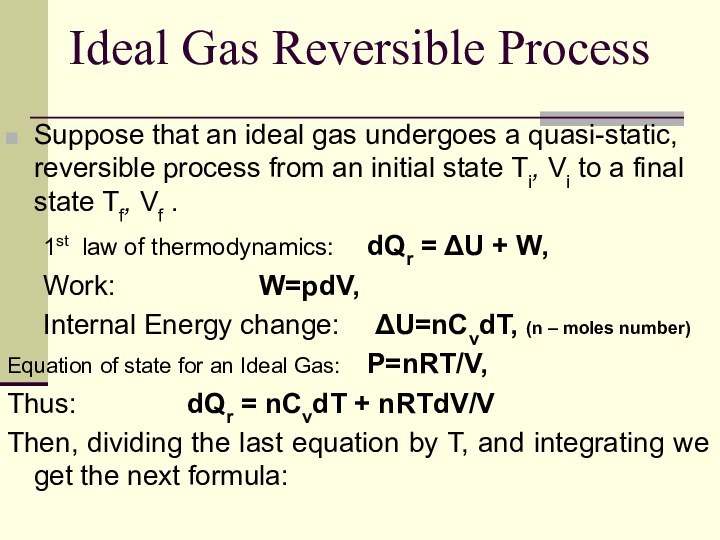
Ideal Gas Reversible Process
Suppose that an ideal gas
undergoes a quasi-static, reversible process from an initial state
Ti, Vi to a final state Tf, Vf .
1st law of thermodynamics: dQr = ΔU + W,
Work: W=pdV,
Internal Energy change: ΔU=nCvdT, (n – moles number)
Equation of state for an Ideal Gas: P=nRT/V,
Thus: dQr = nCvdT + nRTdV/V
Then, dividing the last equation by T, and integrating we get the next formula:
Слайд 24
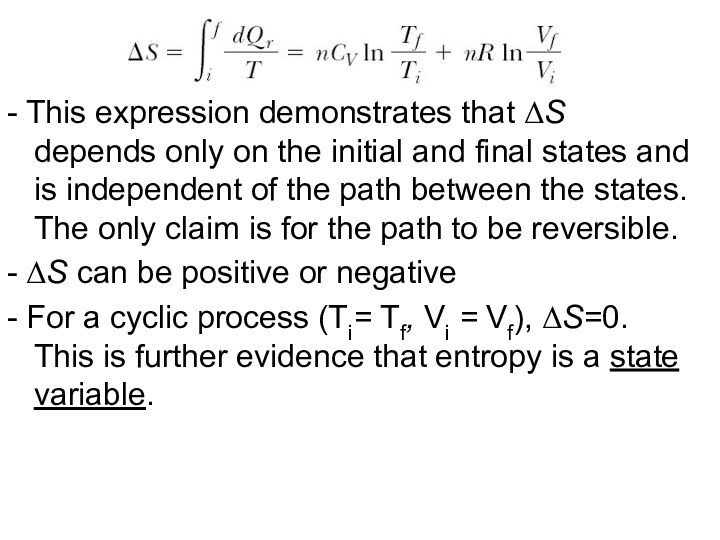
- This expression demonstrates that ΔS depends only
on the initial and final states and is independent
of the path between the states. The only claim is for the path to be reversible.
- ΔS can be positive or negative
- For a cyclic process (Ti= Tf, Vi = Vf), ΔS=0. This is further evidence that entropy is a state variable.
Слайд 25
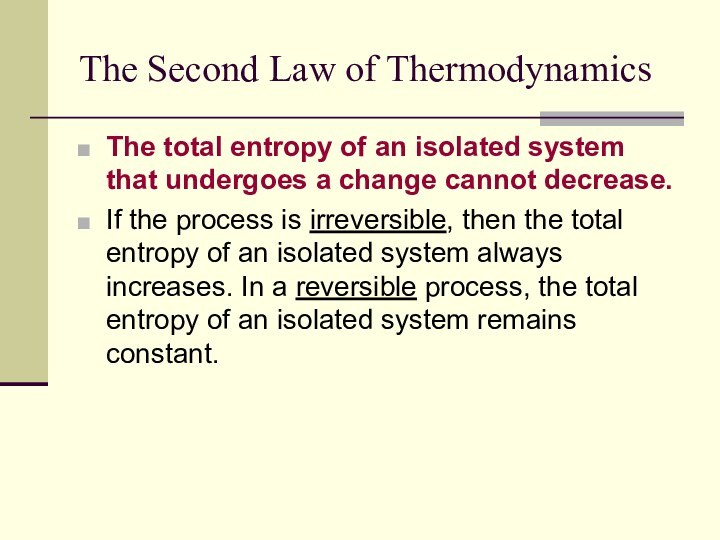
The Second Law of Thermodynamics
The total entropy of
an isolated system that undergoes a change cannot decrease.
If
the process is irreversible, then the total entropy of an isolated system always increases. In a reversible process, the total entropy of an isolated system remains constant.
Слайд 26
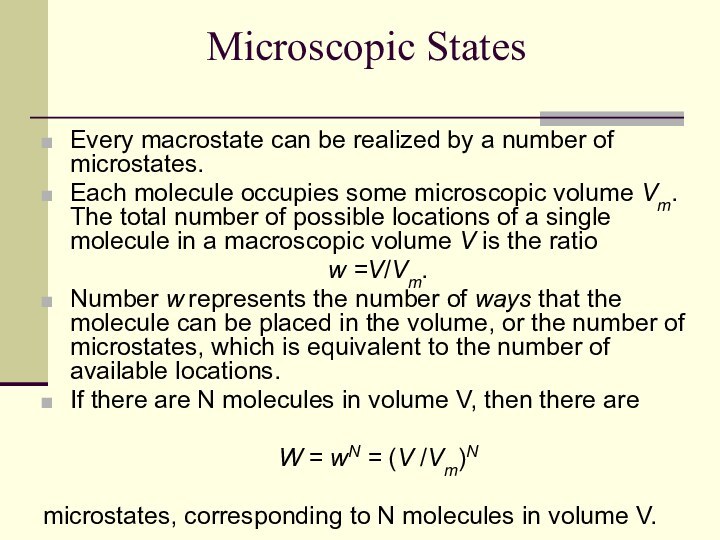
Microscopic States
Every macrostate can be realized by a
number of microstates.
Each molecule occupies some microscopic volume Vm.
The total number of possible locations of a single molecule in a macroscopic volume V is the ratio
w =V/Vm.
Number w represents the number of ways that the molecule can be placed in the volume, or the number of microstates, which is equivalent to the number of available locations.
If there are N molecules in volume V, then there are
W = wN = (V /Vm)N
microstates, corresponding to N molecules in volume V.
Слайд 27
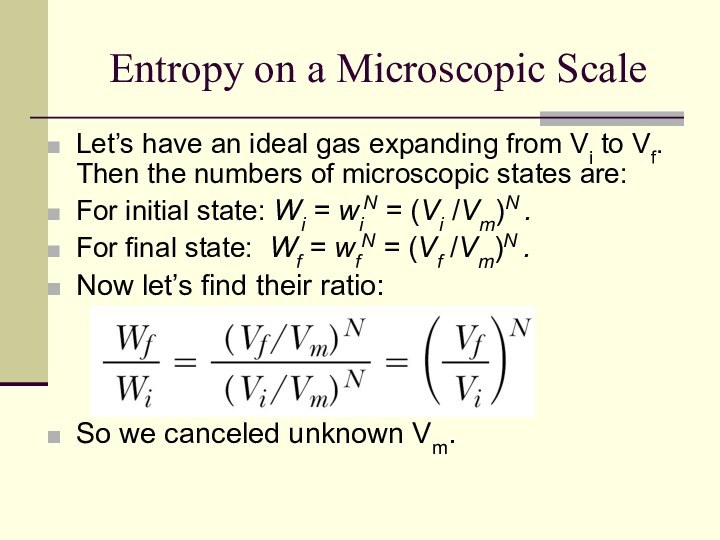
Entropy on a Microscopic Scale
Let’s have an ideal
gas expanding from Vi to Vf. Then the numbers
of microscopic states are:
For initial state: Wi = wiN = (Vi /Vm)N .
For final state: Wf = wfN = (Vf /Vm)N .
Now let’s find their ratio:
So we canceled unknown Vm.
Слайд 28
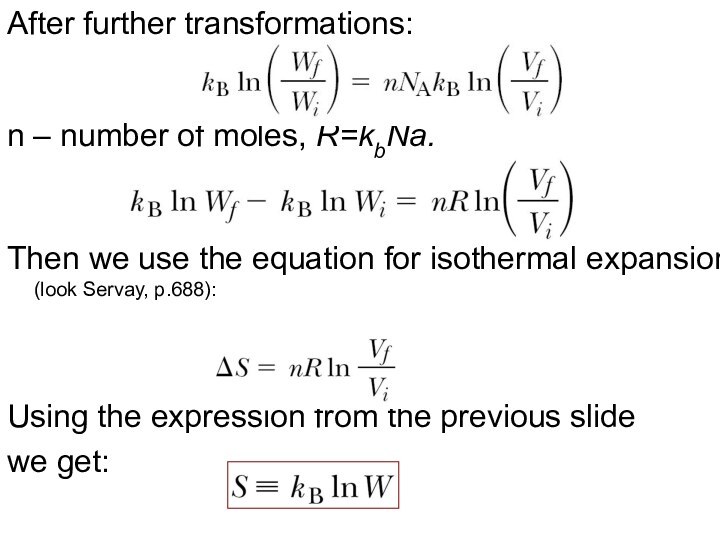
After further transformations:
n – number of moles, R=kbNa.
Then
we use the equation for isothermal expansion (look Servay,
p.688):
Using the expression from the previous slide
we get:
Слайд 29
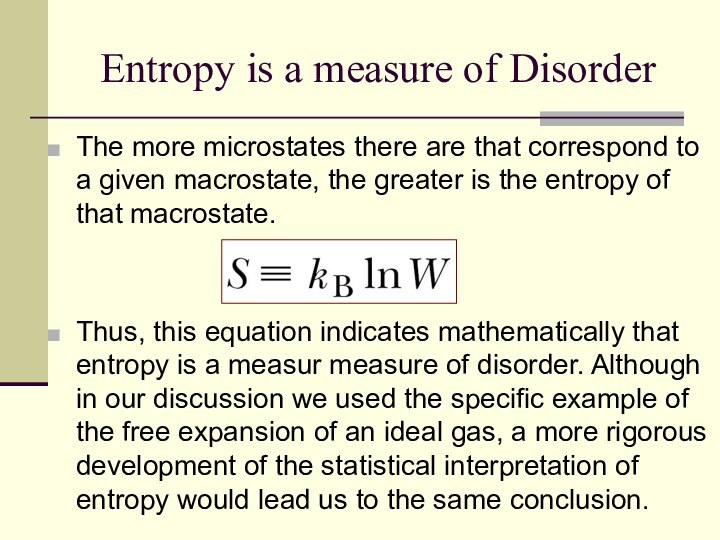
Entropy is a measure of Disorder
The more microstates
there are that correspond to a given macrostate, the
greater is the entropy of that macrostate.
Thus, this equation indicates mathematically that entropy is a measur measure of disorder. Although in our discussion we used the specific example of the free expansion of an ideal gas, a more rigorous development of the statistical interpretation of entropy would lead us to the same conclusion.