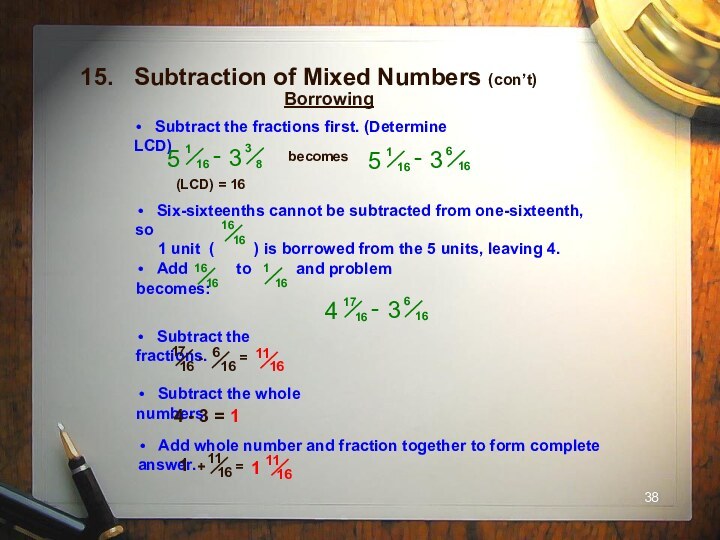
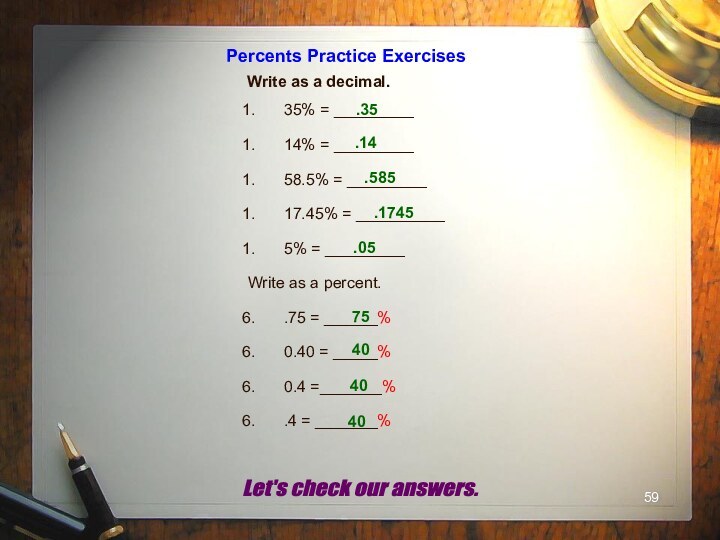
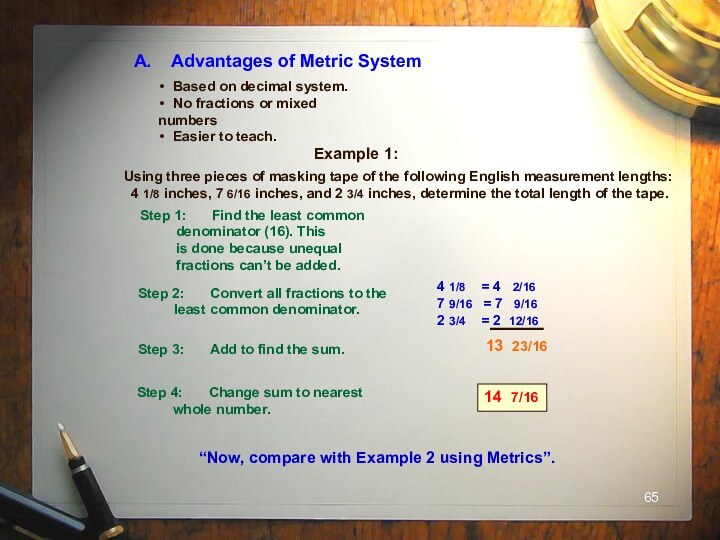
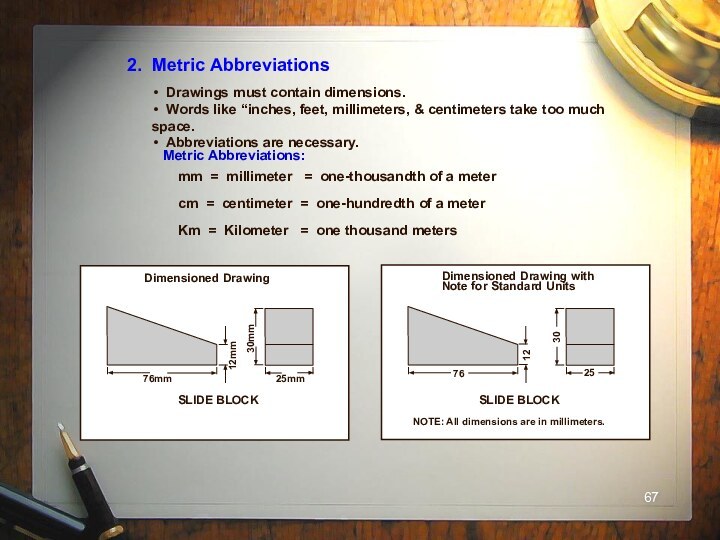
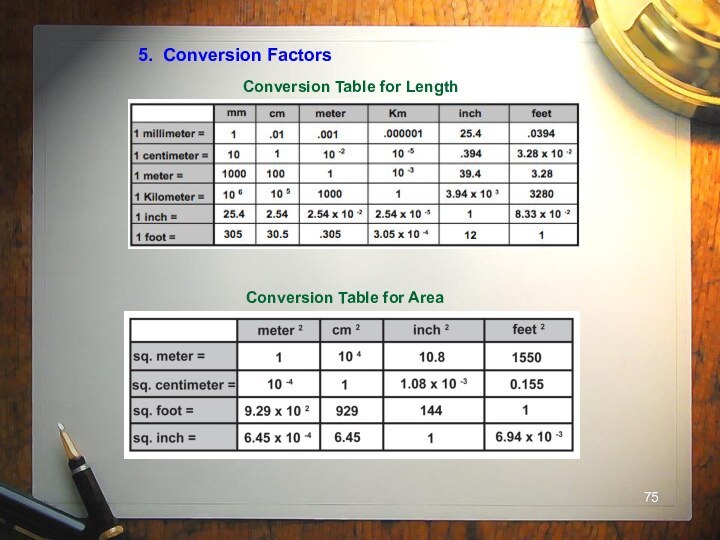
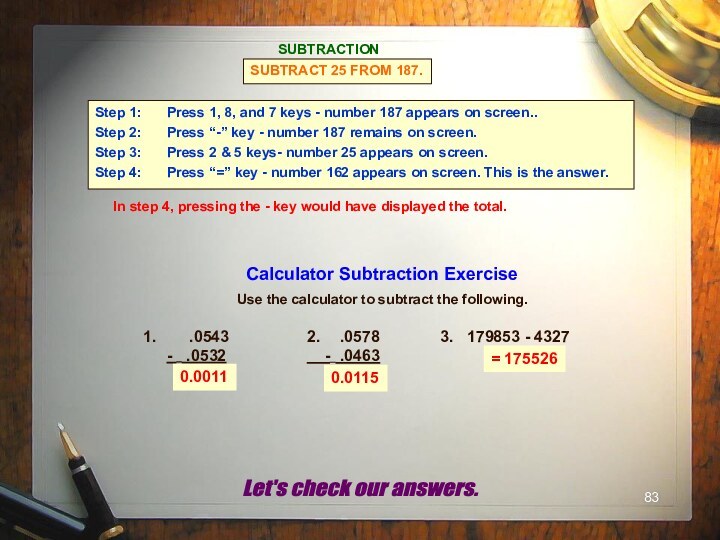
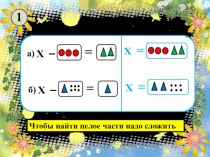
be divided (dividend) inside the division box.
Place divisor
outside.
Move decimal point in divisor to extreme right. (Becomes whole number)
Move decimal point same number of places in dividend. (NOTE: zeros
are added in dividend if it has fewer digits than divisor).
Mark position of decimal point in answer (quotient) directly above decimal
point in dividend.
Divide as whole numbers - place each figure in quotient directly above
digit involved in dividend.
Add zeros after the decimal point in the dividend if it cannot be divided
evenly by the divisor.
Continue division until quotient has as many places as required for the
answer.
Rules For Dividing Decimals