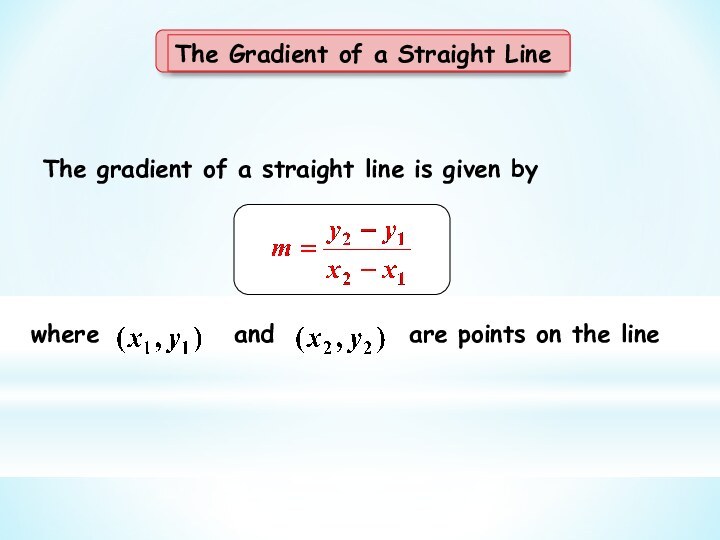
by
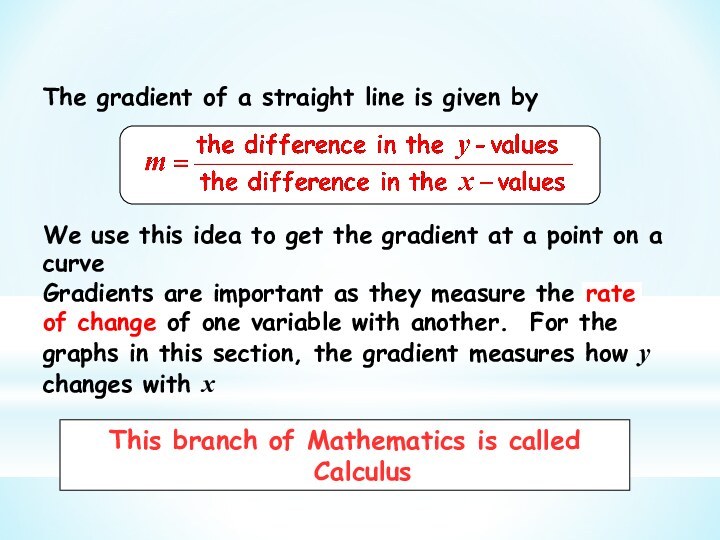
We use this idea to get the gradient at
a point on a curve This branch of Mathematics is called Calculus
FindSlide.org - это сайт презентаций, докладов, шаблонов в формате PowerPoint.
Email: Нажмите что бы посмотреть

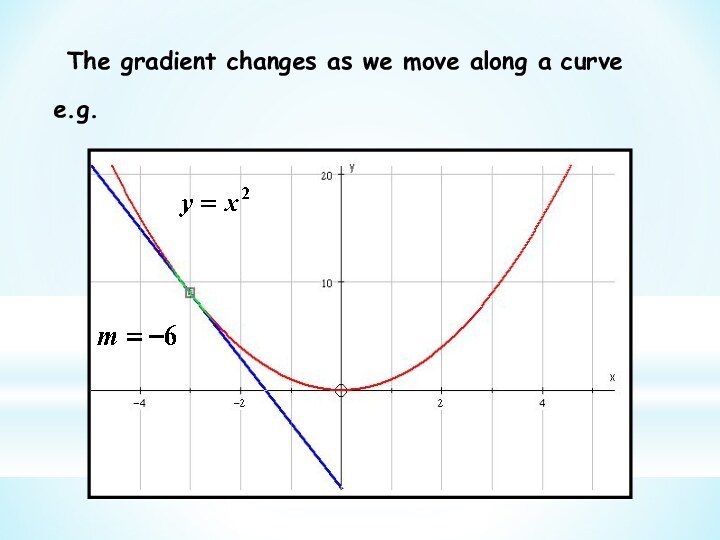
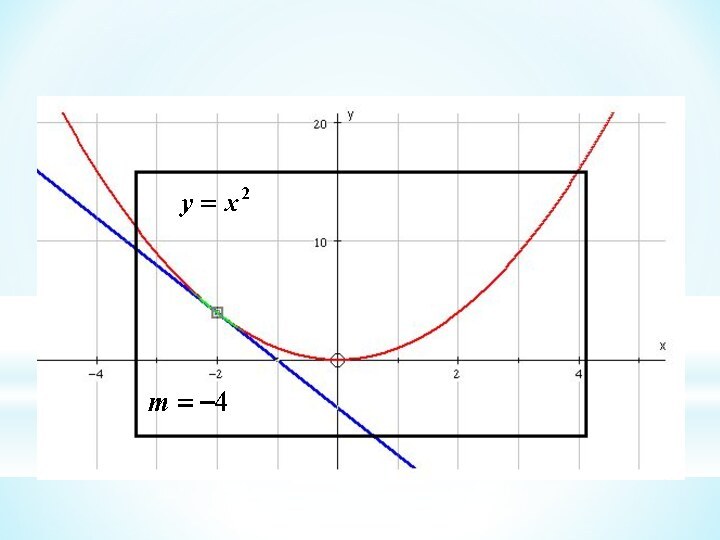
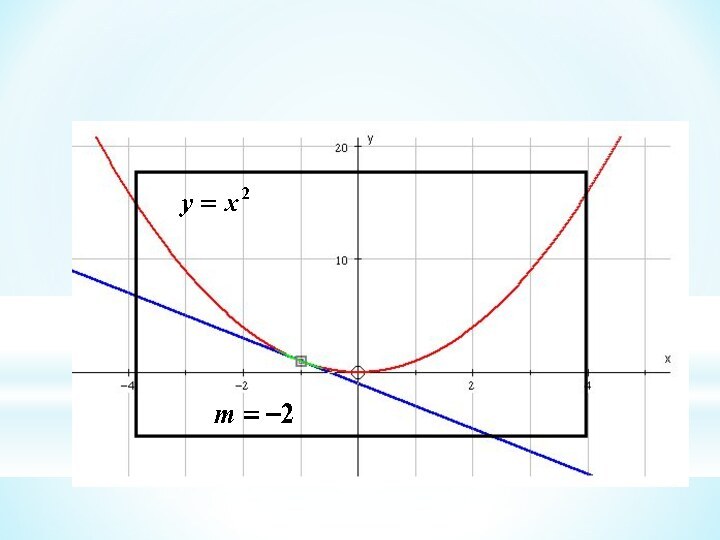
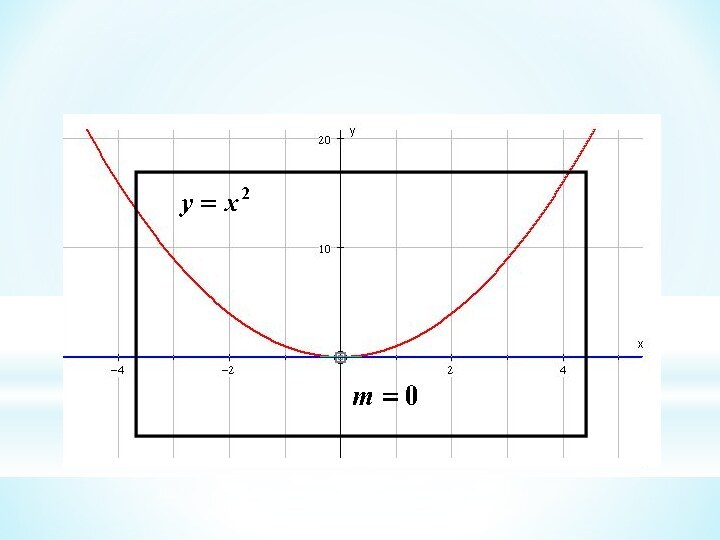

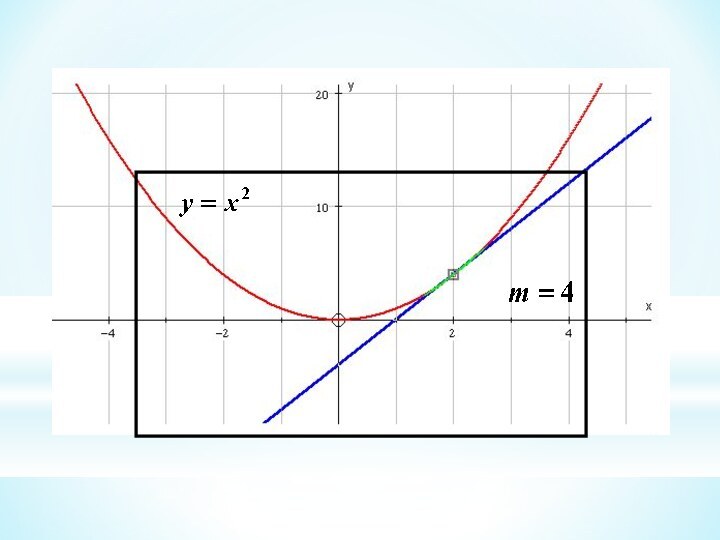
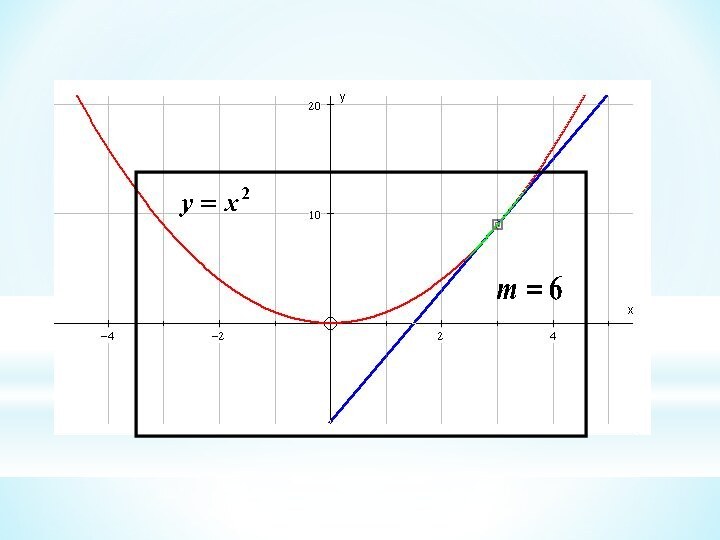
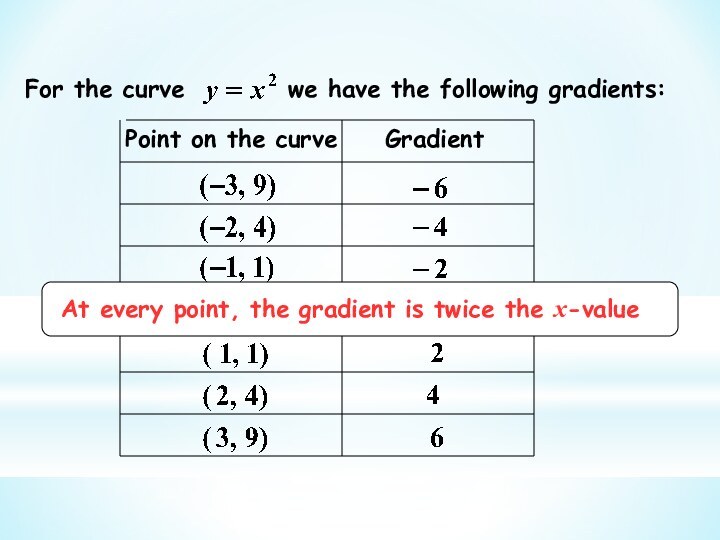
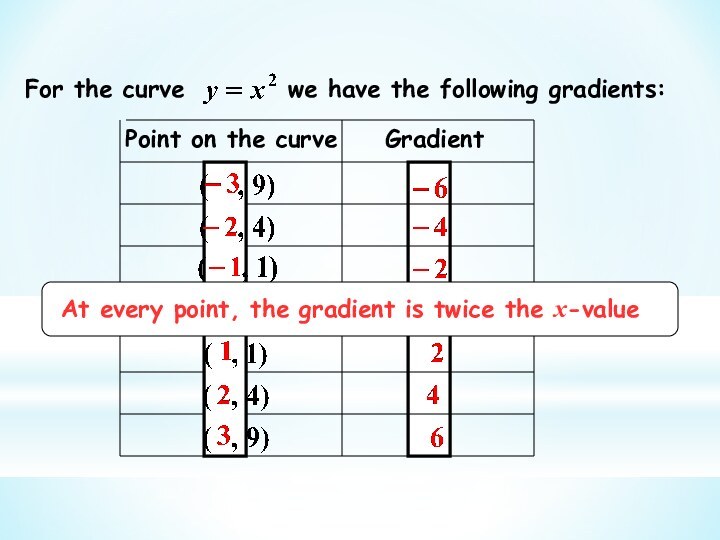
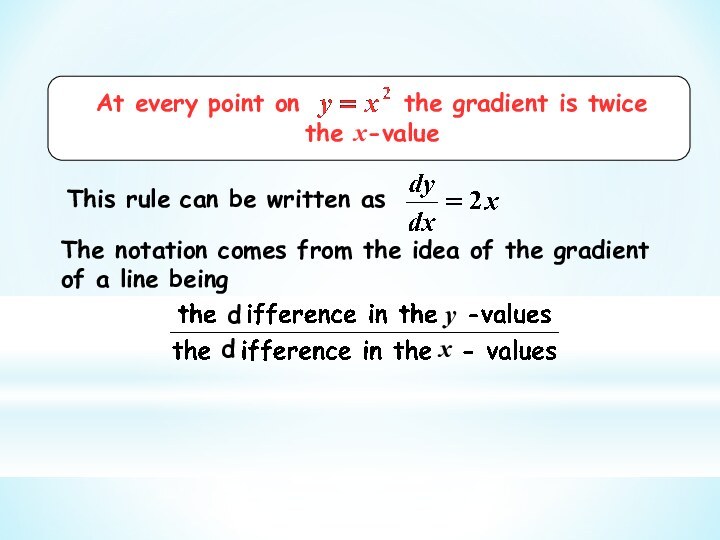

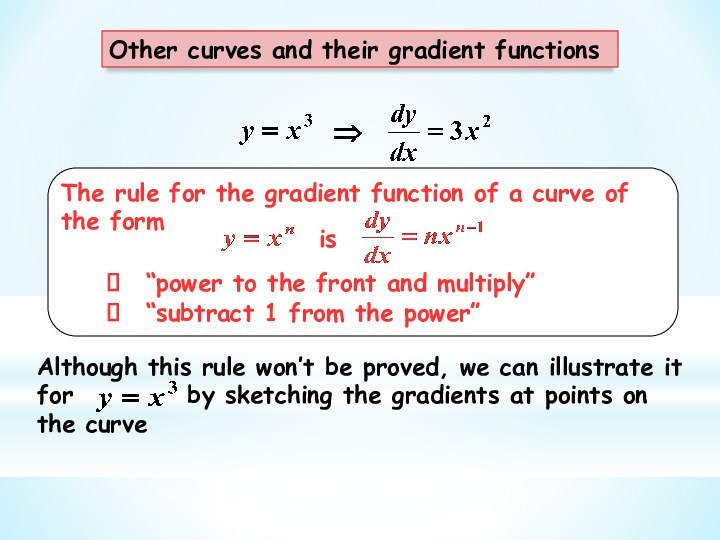
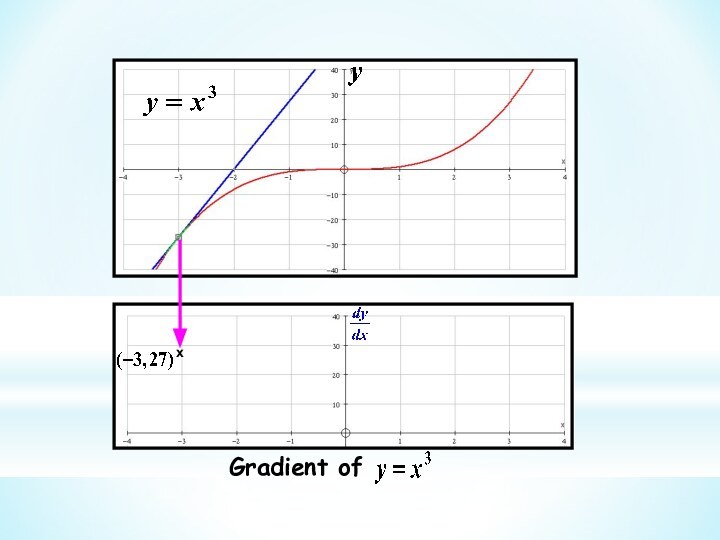
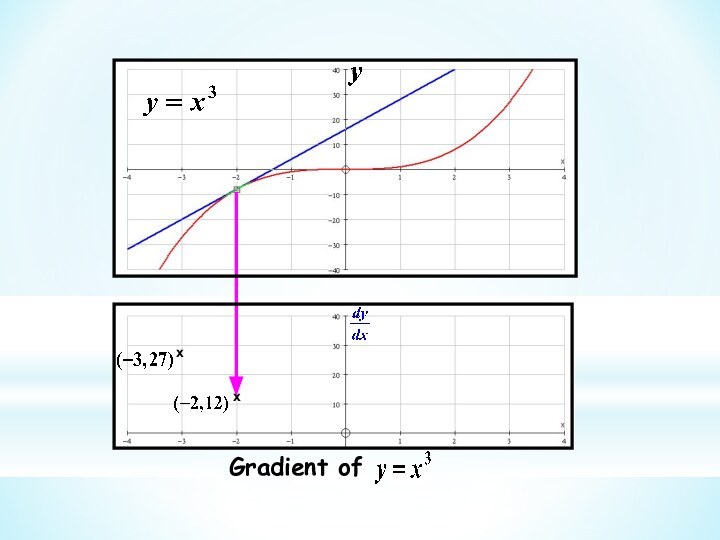
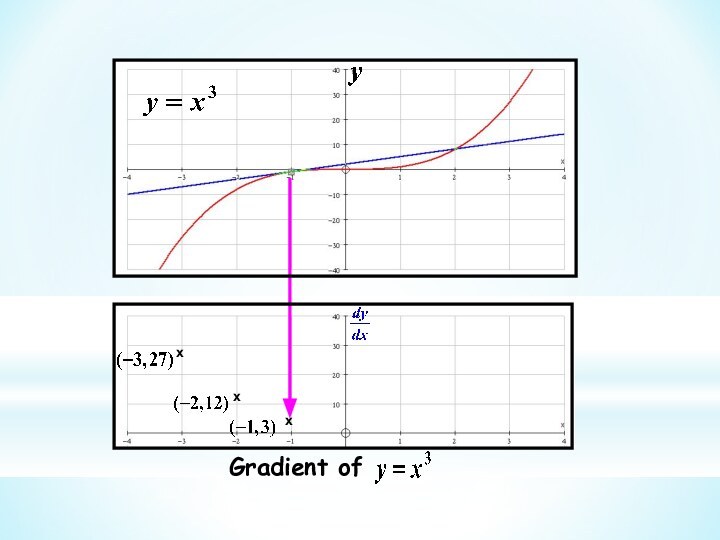
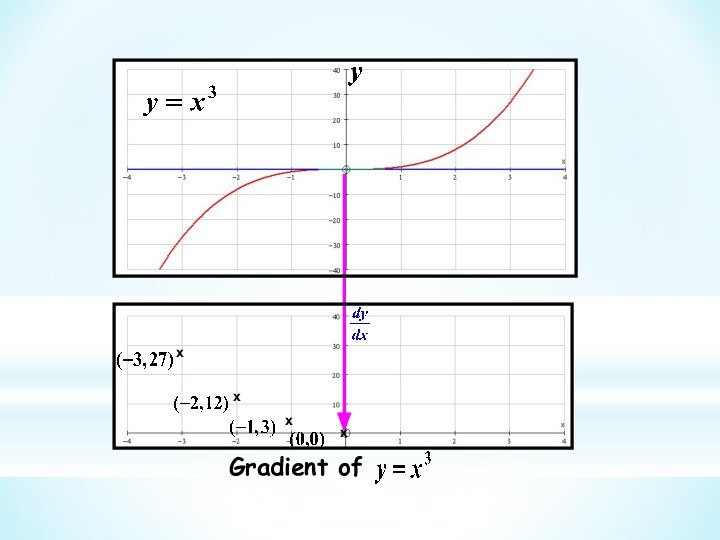
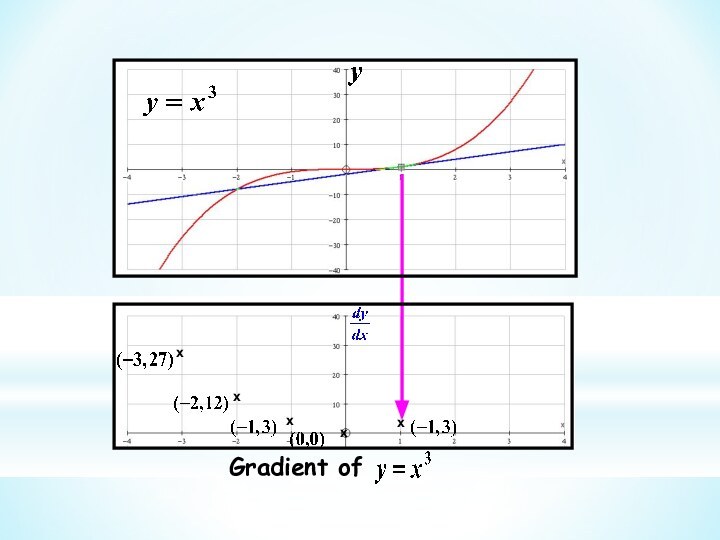
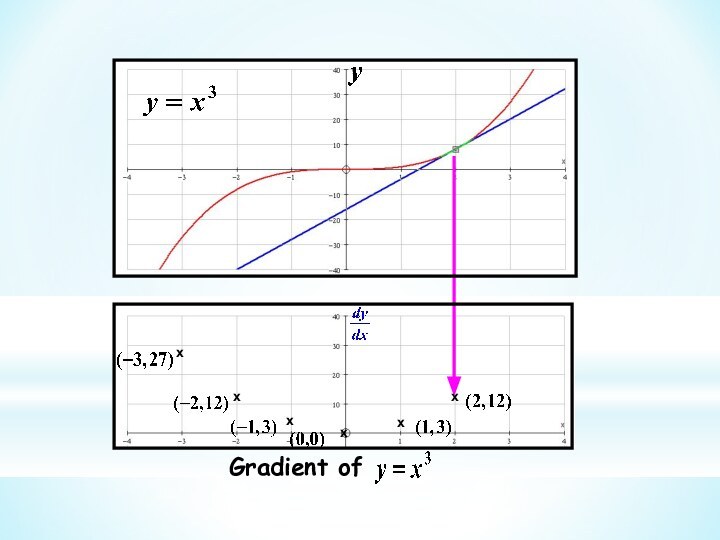
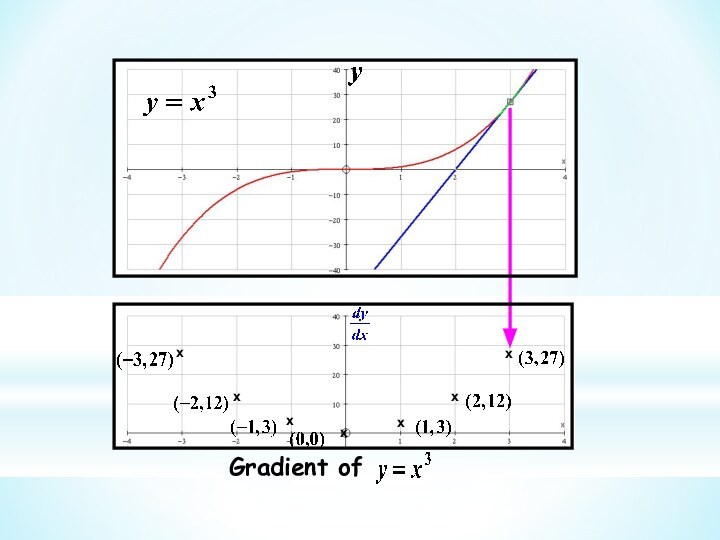
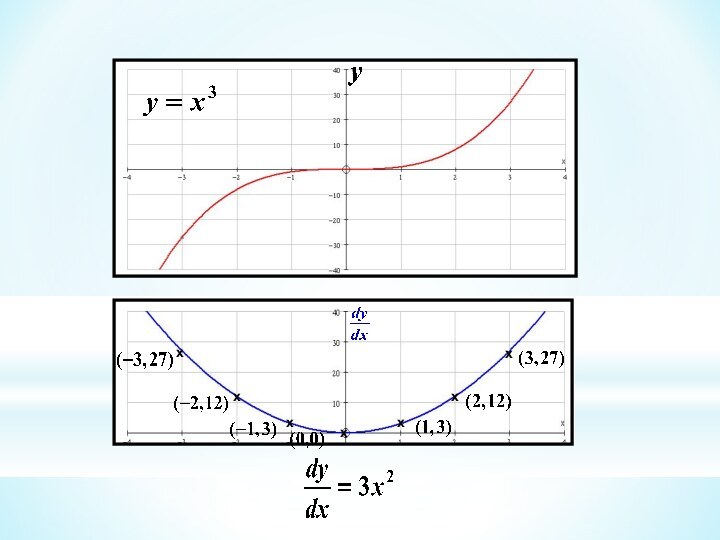

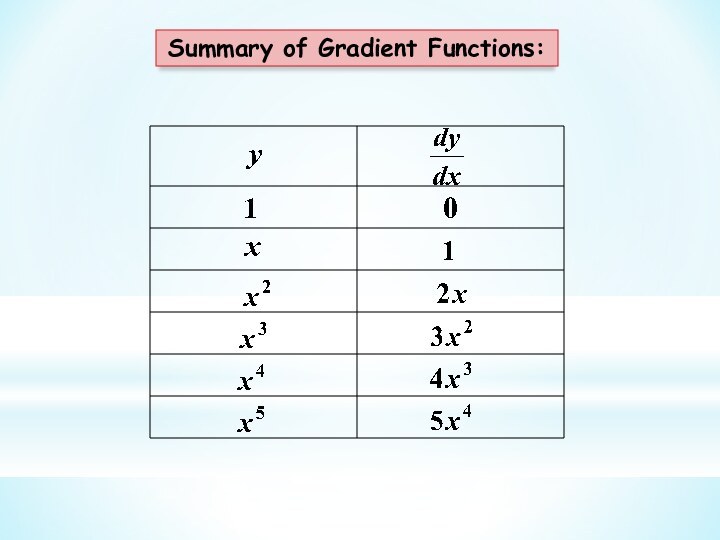
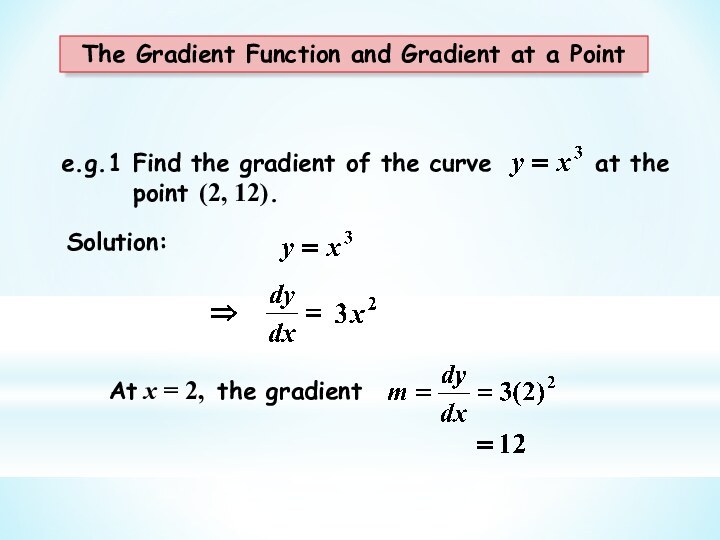
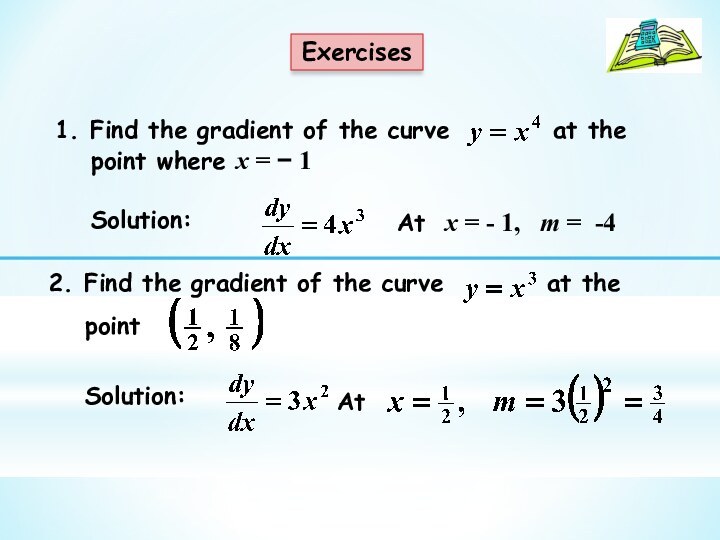
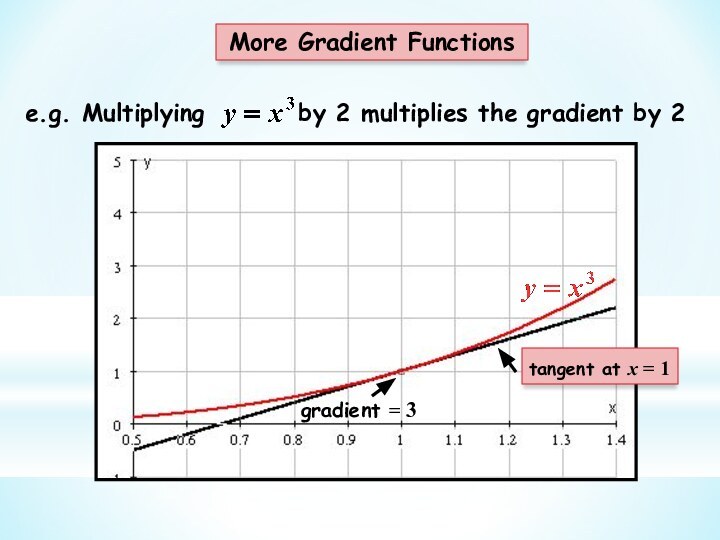
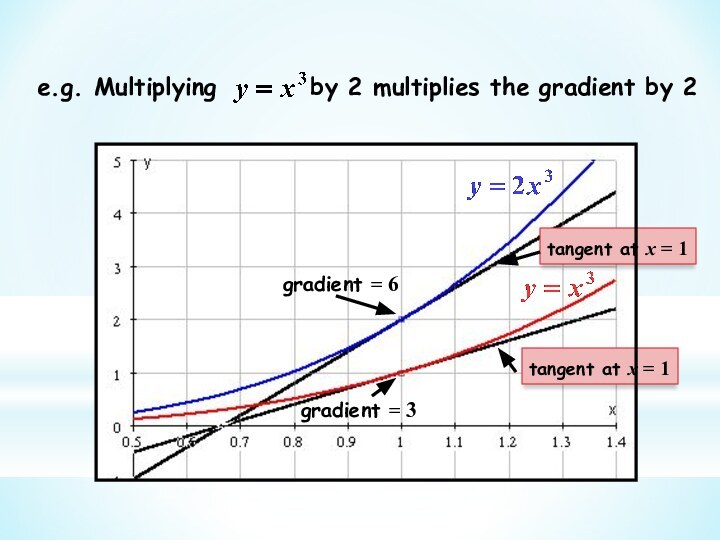
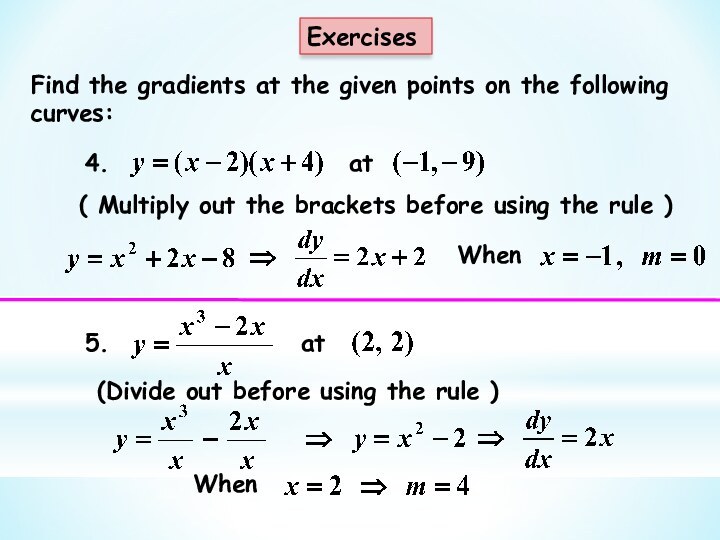
This branch of Mathematics is called Calculus
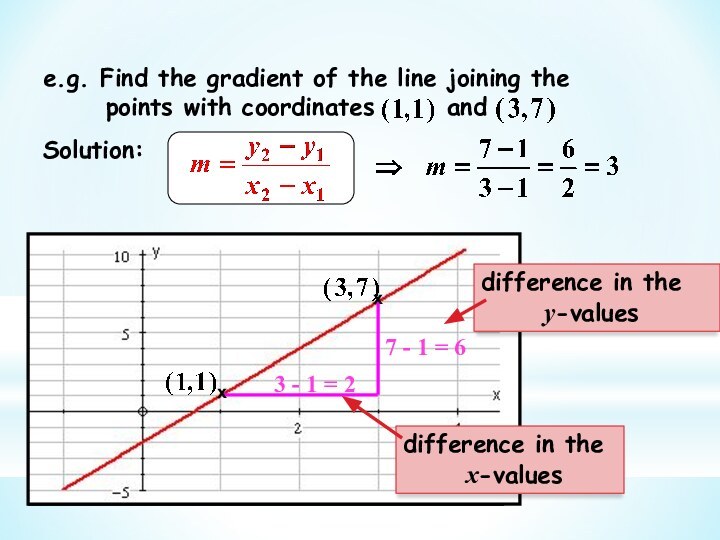
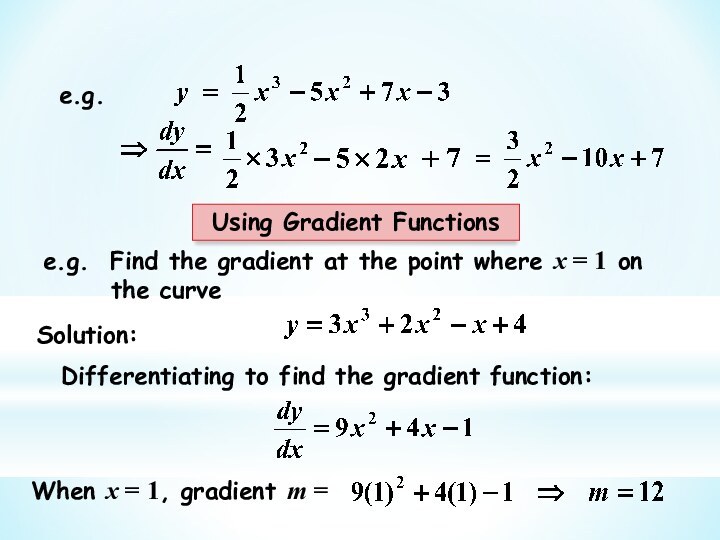
e.g.
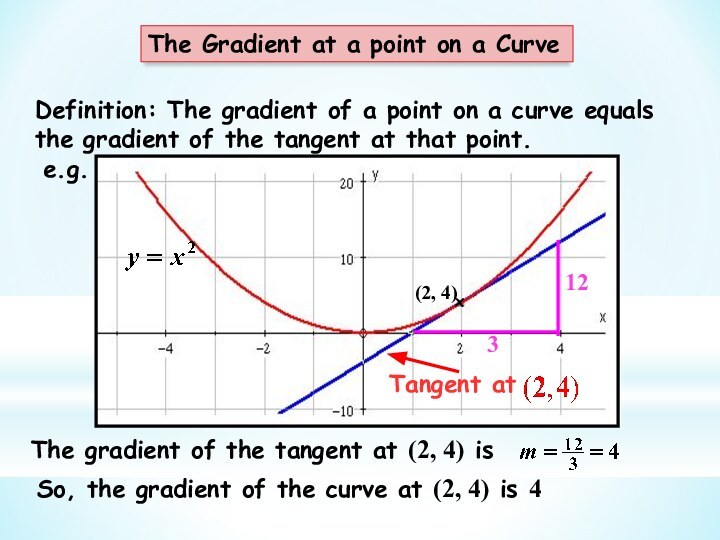
3
12
So, the gradient of the curve at (2, 4) is 4
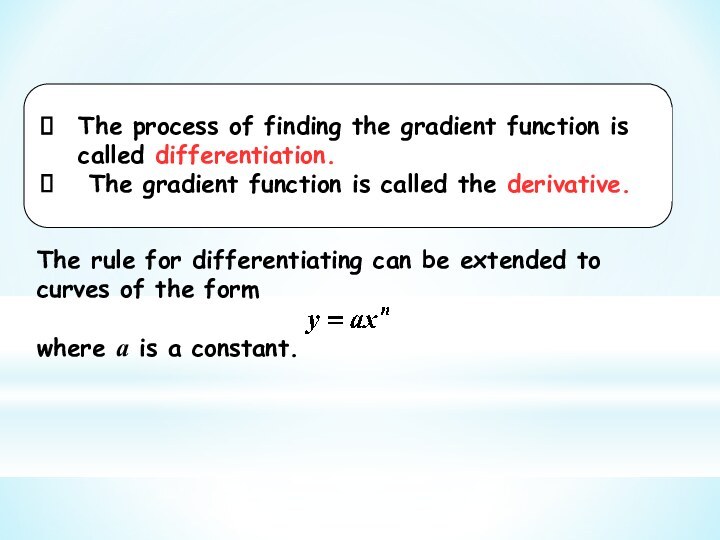
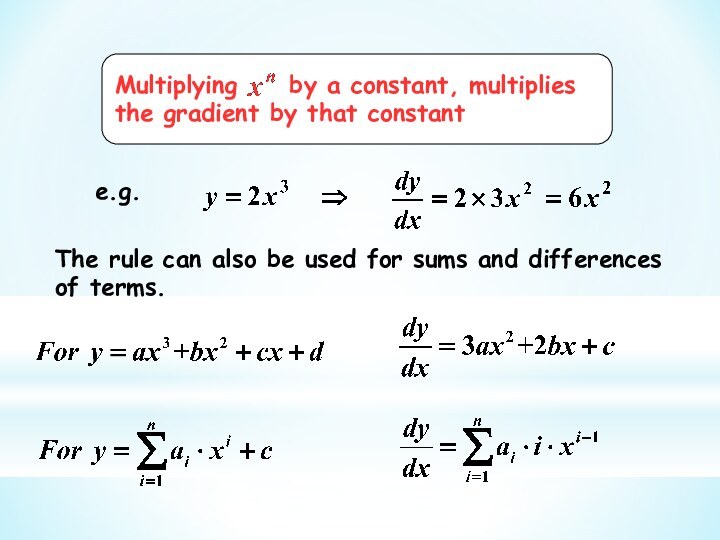
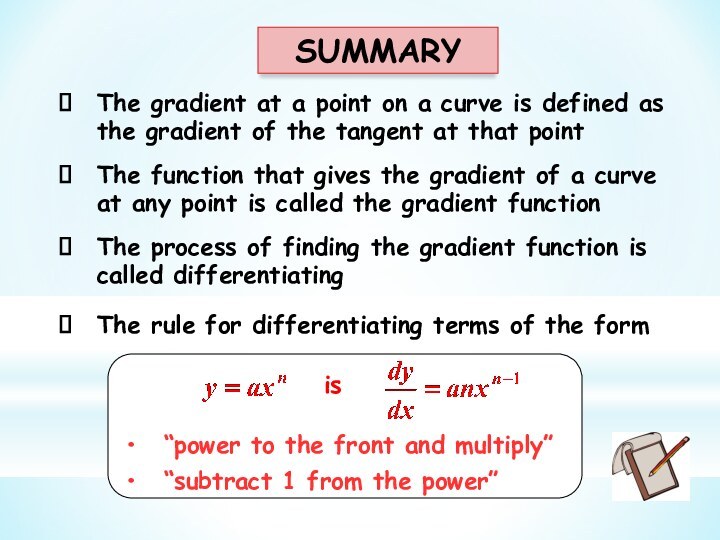
The process of finding the gradient function is called differentiating
The function that gives the gradient of a curve at any point is called the gradient function
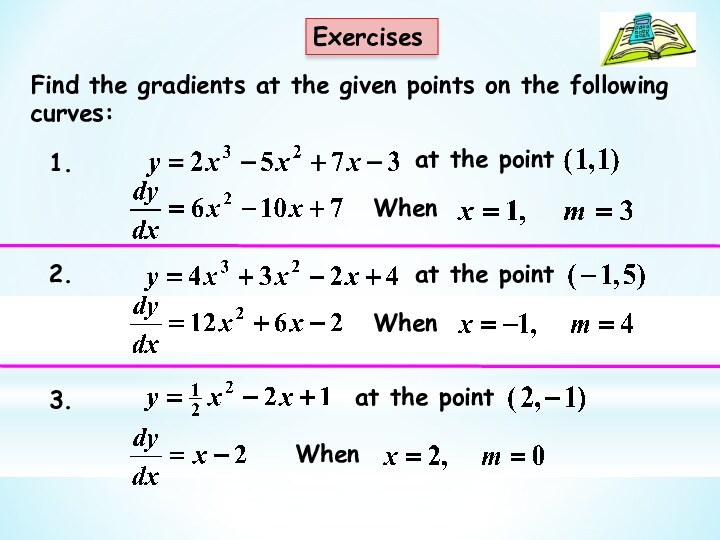
The rule for differentiating terms of the form